You irrational numbers caused great disquiet in mathematicians for a long period. Today, already well defined, we know as an irrational number the one whose decimal representation is always a non-periodic decimal. The main characteristic of irrationals, and what makes them different from rational numbers, is that they cannot be represented by a fraction.
The study of irrational numbers was deepened when, when calculating problems involving the Pythagorean theorem, non-exact roots were found. The act of looking for a solution to these inexact roots made the existence of non-exact tithes remarkable. periodic, that is, of numbers whose decimal part is infinite and does not have a good sequence. defined. The main irrational numbers are non-periodic decimals, non-exact roots, and π.
Read too: Square root - case of rooting where the radical index is 2
Set of irrational numbers
Before the study of irrational numbers, sets of numbers were studied natural, integers and rationals. When delving deeper into the study of the rectangle triangle, it became clear that
there are some roots that have no exact solution, in particular, it was possible to see that non-exact root solutions are numbers known as non-periodic tithes.In the midst of this unrest, many mathematicians have tried to demonstrate, unsuccessfully, that inexact roots are rational numbers and which can be represented as a fraction, but what was realized was that these numbers could not be represented in this form. As, until now, the set of rational numbers did not include these numbers, the need arose to create a new set, known as the set of irrational numbers.
A number is irrational when its decimal representation is a non-periodic decimal. |
What are irrational numbers?
To be an irrational number, it has to satisfy the definition, that is, the its decimal representation is a non-periodic decimal. The main characteristic of non-periodic decimals is that they cannot be represented by means of a fraction, which shows that irrational numbers are the opposite of rational numbers.
The main numbers with this feature are the roots not exact.
Examples:
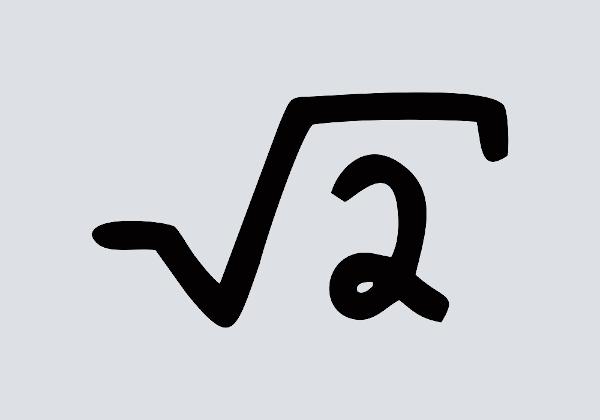
a) √2
b) √5
c) √7
d) √13
When looking for non-exact root solutions, that is, performing the decimal representation of these numbers, always we will find a non-periodic decimal, which makes these numbers elements of the set of irrational.
In addition to non-exact roots, there are non-periodic decimals themselves, for example, if we calculate non-exact roots, we will find a non-periodic decimal.
√2 = 1,41421356...
√5= 2,23606797...
Irrational numbers are commonly represented by Greek letters, because it is not possible to write all its decimal places.
The first one is the π (read: pi), present in the calculation of area and perimeter of circles. Has a value equal to 3,1415926535…
In addition to π, another very common number is ϕ (read: fi). He is found in problems involving the proportion golden. It has a value equal to 1.618033...
See too: What are prime numbers?
Do not stop now... There's more after the advertising ;)
rational and irrational number
When analyzing the number sets, it is important to differentiate between rational numbers and irrational numbers. The union of these two sets forms one of the most studied sets in mathematics, the set of reals, that is, the set of real numbers it is the joining of numbers that can be represented as fractions (rational) with numbers that cannot be represented as fractions (irrational).
In the set of rational numbers, there are the integers, the natural ones, the exact decimals, and the periodic decimals.
Examples of rational numbers:
-60 → integer
2.5 → exact decimal
5.1111111… → periodic decimal
The irrational numbers are non-periodic decimals, so there is no number that is rational and irrational at the same time.
Example of irrational numbers:
1,123149… → non-periodic tithe
2.769235… → non-periodic tithe
Operations with irrational numbers
addition and subtraction
THE addition and the subtraction of two irrational numbers is usually just represented, unless a decimal approximation of these numbers is used, for example:
a) √6 + √5
b) √6 – √5
c) 1.414213… + 3.1415926535…
We can't add or subtract the values because of the radicals, so we've just left the operation indicated.
In decimal representations, it is also not possible to perform the exact sum, so to add two irrational numbers, we need a rational approximation., and this representation is chosen according to the need for accuracy of this data. The more decimal places we consider, the closer to the exact sum we get.
Observation:the set of irrational numbers is not closed to addition or subtraction, this means that the sum of two irrational numbers can result in a number that is not rational. For example, if we calculate the difference of an irrational number by its opposite, we have to:
a) √2 – √2 = 0
b) π + (-π) = 0
We know that 0 is not an irrational number.
Multiplication and division
The multiplication and division of irrational numbers can be done if the representation is a radiciation, however, like addition, in decimal representation, that is, multiplying or dividing two decimals, a rational approximation of this number is required.
a) √7 · √5 = √35
b) √32: √2 = √16 = 4
Note also that, in example b, 4 is a rational number, which means that the multiplication and division of two irrational numbers are not closed, that is, they can have a rational result.
solved exercises
Question 1 - Review the following numbers:
I) 3.1415926535
II) 4,1234510….
III) 2π
IV) 1.123123123...
V) √36
VI) √12
These are irrational numbers:
A) Only I, IV and V
B) Only II, III and VI
C) Only II, IV and VI
D) Only I, II, III and VI
E) Only III, IV, V and VI
Resolution
Alternative B
I → the number is exact decimal, rational.
II → the number is a non-periodic, irrational decimal.
III → π is irrational, and its double, that is, 2π, is also irrational.
IV → the number is a periodic, rational decimal.
V → exact, rational root.
VI → root not exact, irrational.
Question 2 - Please judge the following statements:
I – The set of real numbers is the union of rational and irrational;
II – The sum of two irrational numbers can be a rational number;
III – Tithes are irrational numbers.
Analyzing the statements, we can say that:
A) Only statement I is true.
B) Only statement II is true.
C) Only statement III is true.
D) Only statements I and II are true.
E) All statements are true.
Resolution
Alternative D
I → True, because the definition of the set of real numbers is the union between rational and irrational.
II → True, when we add a number to the opposite of it, we will have as a result the number 0, which is rational.
III → False, non-periodic tithes are irrational.
By Raul Rodrigues de Oliveira
Maths teacher