Some problem situations call for the use of fractional algebraic equations, this type of equation needs to be solved taking into account some restrictions, as we cannot perform divisions by zero. Below we have some problems and their detailed resolutions, so that you can solve all your doubts.
Example 1
R$14,000.00 should be distributed equally to a certain number of people. Before the distribution was made, 10 people left, and it was only necessary to distribute R$ 12,000.00 so that each one would receive the same amount they would have received at the beginning. What was the number of people initially?
Equating the equation, we have: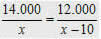
(Multiply the numerator of the 1st fraction by the denominator of the 2nd fraction and the numerator of the 2nd fraction by the denominator of the 1st)
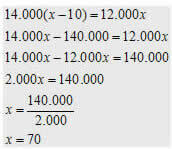
Example 2
Carlos performed a job in 8 days. Mario performed the same job in x days. Together they performed the same job in 3 days. Determine the value of x.
Equating the equation we have: 
Example 3
A vehicle with an average speed travels 4000 km that separate city A from city B in x hours. Another vehicle, with the same average speed as the first, travels the 2200 km that separate city C from city D in (x – 12) hours. Determine the value of x.
We calculate the average speed of a mobile by dividing the space traveled by it by the time spent on the journey.
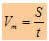, where S: space variation and t: travel time.
Do not stop now... There's more after the advertising ;)
Equating the equation, we have:
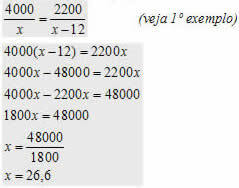
by Mark Noah
Graduated in Mathematics
Brazil School Team
Equations - Math - Brazil School
Would you like to reference this text in a school or academic work? Look:
SILVA, Marcos Noé Pedro da. "Problem Solving Involving Fractional Equations"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/resolucao-problemas-envolvendo-equacoes-fracionarias.htm. Accessed on June 29, 2021.
