Calculations related to areas of regular plane figures are somewhat easily performed due to existing mathematical formulas. In the case of figures such as triangle, square, rectangle, trapezoids, diamonds, parallelograms, among others, it is enough to relate the formulas to the figure and perform the necessary calculations. Some situations require auxiliary tools to obtain areas, such as regions under a curve. For such situations we use calculations involving the notions of integration developed by Isaac Newton and Leibniz.
We can algebraically represent a curve in the plane through a formation law called a function. The integral of a function was created in order to determine areas under a curve in the Cartesian plane. Calculations involving integrals have several applications in Mathematics and Physics. Note the following illustration:

To calculate the area of the demarcated region (S) we use the integrated function f on the variable x, between the range a and b:

The main idea of this expression is to divide the demarcated area into infinite rectangles, because intuitively the integral of f (x) corresponds to the sum of the rectangles of height f (x) and base dx, where the product of f (x) by dx corresponds to the area of each rectangle. The sum of the infinitesimal areas will give the total surface area under the curve.

When solving the integral between limits a and b, we will have the following expression as a result:

Example
Determine the area of the region below delimited by the parabola defined by the expression f (x) = – x² + 4, in the range [-2.2].

Determining the area through function integration f (x) = –x² + 4.
For this we need to remember the following integration technique:
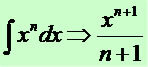

Therefore, the area of the region delimited by the function f (x) = –x² + 4, ranging from -2 to 2, it is 10.6 area units.
by Mark Noah
Graduated in Mathematics
Brazil School Team
Roles - Math - Brazil School
Source: Brazil School - https://brasilescola.uol.com.br/matematica/area-sob-uma-curva.htm
