You numbers they accompany the primitive human needs to quantify, count and measure. Because of these needs, it became essential to create the idea of numbers and also symbols that would represent them through writing.
Throughout history, several civilizations developed the notion of numbers and used, many times, the body itself to represent this and do counts, until it was possible to portray the numbers via different symbols in order to represent them from written form. Today we use the ind numeralsO-Arabics,which allow us to indicate any number using ten different symbols {0, 1, 2, 3, 4, 5, 6, 7, 8, 9}.
With the development of society - and, consequently, of mathematics -, numerical sets emerged throughout history. Are they:
natural numbers;
integers;
rational numbers;
irrational numbers;
real numbers.
Read too: Decimal numbering system — the numbering system we use
Summary about numbers
The notion of number was developed to meet man's need to count and measure.
Throughout history, different peoples have developed different numbers.
The numbers we use today are divided into sets of numbers, namely: natural numbers, integers, rational numbers, irrational numbers, and real numbers.
Do not stop now... There's more after the advertising ;)
What are numbers?
the numbers are primitive objects of mathematics that serve to indicate order, measure or quantity. We do not know for sure when man developed the notion of quantity and, as a consequence, the notion of numbers.
The concept of number, then, accompanies the development of humanity, and today numbers are represented by the symbols {0, 1, 2, 3, 4, 5, 6, 7, 8, 9} in our society, but there have been several other systems of numbering. Numbers are elements that underlie mathematics and can be expressed by sound, in our speech, or by writing.
history of numbers
The concept of number emerges in humanity from the moment the need to count food and objects. Therefore, during the existence of cavemen, the notion of numbers was already necessary to count, for example, the amount of fish caught.
Over time, with the development of agriculture, numbers were again necessary, so that it was possible to count the amount of collected fruits or animals in a herd.
Thus, over the years, society was changing, and human beings realized how much it was necessary to development ofThe writing. With the development of writing by the Sumerians, the first figures for the representation of numbers also appeared. There are records of other peoples who developed numbering systems, such as the Egyptians, the Mayans, the Chinese and the Hindus.
Currently, we use the ind numbering systemO-Arabic,which has base 10 and allows us, with ease, to carry out operations between two numbers. As the need for mathematics that man mastered in everyday life increased, numerical sets emerged.
Read too: What are prime numbers?
Numerical sets
You numerical sets have been emerging throughout history to meet new demands of the population. The first numerical set known to us is the set of natural numbers, and there are others, such as the set of whole numbers, the set of rational numbers, the set of irrational numbers, and finally, the set of real numbers.
Set of natural numbers (N)
You natural numbers were the first to be used by human beings.snot the integers and positives, which we use in our daily lives to count and sort.
N = {0, 1, 2, 3, 4, 5, 6…}
The set of natural numbers has infinite elements. Each number always has a well-defined successor, because to find the successor of a natural number just add 1 to this number.
Set of integers (Z)
the set of whole numbers is an expansion of the set of natural numbers, as every natural number is an integer too. This set is created from the human need to represent negative numbers. Today it is quite common to see negative numbers in temperature measurements, for example. The integers are:
Z = {…– 4, – 3, – 2, – 1, 0, 1, 2, 3, 4,…}
O set of integers is also infinite, but for both sides, that is, there are infinite negative and positive numbers.
Set of rational numbers (Q)
the set of rational numbers arises from the need for more accurate measurements. It was not always possible to represent a measure using whole numbers. It was then that the precision of the existence of decimal numbers and also of fractions.
So the set of rational numbers is also an enlargement of whole numbers, that is, every whole number is rational, but what changes is that there is an increase in the numbers that can be represented by fractions.
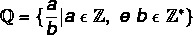
It is impractical to represent the set of these numbers in a list, as in the previous cases, because the numbers rationals can be expressed as a fraction, which makes the decimal numbers also integrate this set. So, as much as we have a well-defined order relationship, that is, we know which number is higher or lower when compared, still it is not possible to define who is the successor of a given number in the set of rational numbers.
Irrational numbers (I)
You irrational numbers they are not an extension of the previous sets, but a new numerical set. During the resolution of certain problems, the result found was an inexact root and, from then on, there was a need for a new set.
irrational numbers are composed of inexact roots and also non-periodic tithes. Furthermore, a number will never be rational and irrational at the same time, since to be irrational the number cannot be expressed as a fraction. The number √2, for example, is irrational because its square root is not exact, generating a non-periodic decimal.
Real numbers (R)
the set of real numbers is nothing but the unity dthe irrational numbers and dthe rational numbers, forming a new set, which is currently the most used in the study of functions, among other topics.
Video lesson on numerical sets
other numbers
Set of complex numbers (C)
In addition to the sets presented, there is also the set of complex numbers (Ç). This is a classification made for deeper mathematics studied by experts. Although less common, complex numbers are of great importance. We know as complex numbers the roots of negative numbers.We denote i = √– 1 to represent any complex number. For example, 1 + √– 4 is represented by 1 + 2i.
Read too: Fun Facts About Dividing Natural Numbers
Solved Exercises on Numbers
Question 01
About numbers, we know that they are divided into sets, known as number sets. Based on this knowledge, judge the following statements:
I → Every irrational number is a real number.
II → Every rational number is an integer.
III → Every irrational number is a rational number.
Mark the correct alternative:
A) Only I is true.
B) Only II is true.
C) Only III is true.
D) All are false.
Resolution:
Alternative A
I → True, because the set of real numbers is formed by the union of rationals with irrationals.
II → False, as there are numbers that are rational and that are not integers.
III → False, as a number cannot be irrational and rational at the same time.
question 02
About the invention of numbers, judge the following statements:
A) Numbers are a modern creation, because when men were nomads, it was not necessary to use numbers, as they were only occupied with hunting and fishing. So, the notion of number only came up with agriculture.
B) Numbers were invented by men from the advent of commerce, as they needed to make fair exchanges. Before that, there is no record of the use of numbers by men.
C) The numbers were invented by man when he stopped being a nomad and started to raise herds and dedicate himself to plantations, helping to control the cycles of his crops.
D) Although the numbering system we use was not the first to be invented, the idea of number it has accompanied man since the time of caves, with the need to account for the amount of food, among others applications.
Resolution:
Alternative D
The alternative that best describes the history of the invention of numbers is alternative D.
By Raul Rodrigues de Oliveira
Maths teacher