The line segment is defined as a part of the straight, which is delimited by a colon.
Line segments are usually represented within square brackets (Line Segment [AB]) or with a dash above the letters:

Straight, Straight Segment and Semi-straight
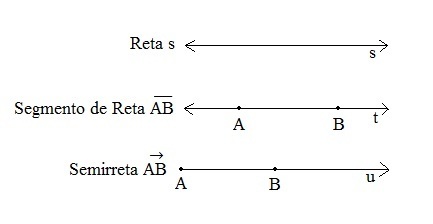
Remember that the straight they are infinite uncurved lines and are therefore represented by arrows on both sides. They are indicated by lowercase letters (r, s, t).
Line segments, on the other hand, are delimited by two distant points within the line, which are indicated by capital letters. In the figure above, points A and B are called the ends of the line segment.
On the other hand, the semi-straight they are unlimited in a sense, since they have a point of origin, however, they do not have a point that delimits their end.
Therefore, the ray is a part of the straight line limited by a point. For this reason, they have an arrow that indicates the direction in which it is infinite.
Know more about:
- Geometric Shapes
- Parallel Lines
- Distance between two points
- Line Equation
- plane geometry
- Spatial Geometry
Types of Line Segments
According to position that occupy the plane, the straight segments are classified into:
Consecutive Segments: when they have a common point. In the figure below, the common point is D.
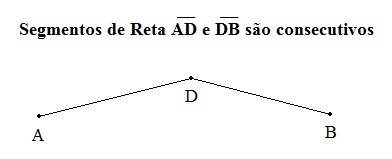
Collinear Segments: when the points belong to the same line. That is, when two or more distinct points share the same line.

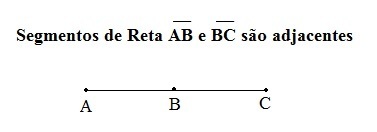
Adjacent Segments: when they are consecutive and linear. That is, they have points in common and a single straight line passes through them.
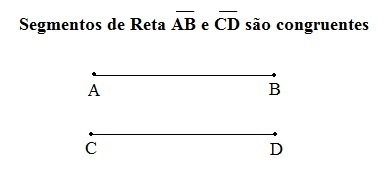
Congruent Segments: when two segments have the same measurement. In the figure below AB~CD (It reads: segment AB is congruent to segment CD).
Midpoint of the Line Segment
The midpoint of a line segment defines the middle of the segment.
In the example below we can see that M is the midpoint of the line segment AB, where AM~MB (It reads: segment AM is congruent to segment MB).

Solved Exercises
1. How many line segments does a cube have?
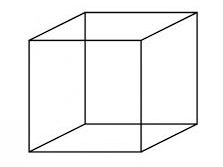
a) 4
b) 8
c) 10
d) 12
e) 14
By analyzing the figure, we can conclude that the cube has 12 corners, which are called edges. The edges, in turn, are straight segments.
Therefore, the cube has 12 straight segments.
Answer: letter D.
2. How many line segments are there in the tetrahedron and the triangle?
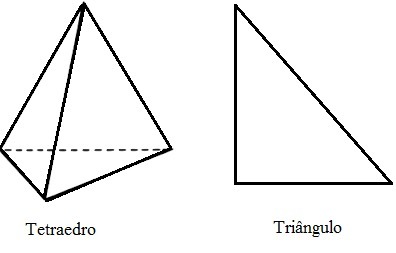
a) 6 and 3
b) 9 and 3
c) 10 and 3
d) 12 and 6
e) 14 and 6
The triangle is a flat figure formed by 3 sides. Each side is considered a straight line. The tetrahedron is a spatial geometric figure composed of 4 triangular faces and 6 edges. Therefore, the tetrahedron has 6 straight segments.
Answer: letter A.