At first degree equations are math sentences like ax + b = 0, where a and b are real numbers and x is the unknown (unknown term).
Several types of problems are solved through this calculation, so knowing how to solve a 1st degree equation is fundamental.
Take advantage of the commented and solved exercises to exercise this important math tool.
question 1
(CEFET/RJ - 2nd phase - 2016) Carlos and Manoela are twin brothers. Half of Carlos' age plus a third of Manoela's age is equal to 10 years. What is the sum of the ages of the two brothers?
Correct answer: 24 years.
As Carlos and Manoela are twins, their ages are the same. Let's call this age x and solve the following equation:
Therefore, the sum of the ages is equal to 12 + 12 = 24 years.
question 2
(FAETEC - 2015) A package of the Tasty biscuit costs R$ 1.25. If João bought N packages of this cookie spending R$ 13.75, the value of N is equal to:
a) 11
b) 12
c) 13
d) 14
e) 15
Correct alternative: a) 11.
The amount spent by João is equal to the number of packages he bought times the value of 1 package, so we can write the following equation:
Therefore, the value of N is equal to 11.
question 3
(IFSC - 2018) Consider the equation , and tick the CORRECT alternative.
a) It is a function of the first degree, its solution is = −1 and its solution set is = {−1}.
b) It is a rational equation, its solution is = −4 and its solution set is = {−4}.
c) It is an equation of the first degree, its solution is = +4 and its solution set is = ∅.
d) It is a second degree equation, its solution is = −4 and its solution set is = {−4}.
e) It is an equation of the first degree, its solution is = −4 and its solution set is = {−4}.
Correct alternative: e) It is an equation of the first degree, its solution is = −4 and its solution set is = {−4}.
The indicated equation is an equation of the first degree. Let's solve the indicated equation:
Therefore, is an equation of the first degree, its solution is = −4 and its solution set is = {−4}.
question 4
(Colégio Naval - 2016) In the exact division of the number k by 50, a person, absently, divided by 5, forgetting the zero and, thus, found a value 22.5 units higher than expected. What is the value of the tens digit of the number k?
to 1
b) 2
c) 3
d) 4
e) 5
Correct alternative: b) 2.
By writing the problem information in the form of an equation, we have:
Therefore, the value of the tens digit of the number k is 2.
question 5
(Colégio Pedro II - 2015) Rosinha paid R$67.20 for a blouse that was being sold at a 16% discount. When her friends found out, they rushed to the store and had the sad news that the discount was over. The price found by Rosinha's friends was
a) BRL 70.00.
b) BRL 75.00.
c) BRL 80.00.
d) BRL 85.00.
Correct alternative: c) R$ 80.00.
Calling x the amount paid by Rosinha's friends, we can write the following equation:
Therefore, the price found by Rosinha's friends was R$ 80.00.
question 6
(IFS - 2015) A Teacher spends of your salary with food,
with housing and still have R$ 1,200.00. What is this teacher's salary?
a) BRL 2,200.00
b) BRL 7,200.00
c) BRL 7,000.00
d) BRL 6,200.00
e) BRL 5,400.00
Correct alternative: b) BRL 7,200.00
Let's call the teacher's salary value x and solve the following equation:
Therefore, this teacher's salary is R$7,200.00.
question 7
(Apprentice Sailor - 2018) Analyze the following figure.
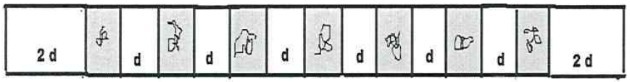
An architect intends to fix on a panel 40 m long horizontally seven engravings 4 m long horizontally each. The distance between two consecutive engravings is d, while the distance from the first and last engraving to the respective sides of the panel is 2d. Therefore, it is correct to say that d it's the same as:
a) 0.85 m
b) 1.15 m
c) 1.20 m
d) 1.25 m
e) 1.35 m
Correct alternative: c) 1.20 m.
The total length of the panel is equal to 40 m and there are 7 engravings with 4 m, so, to find the measure that will be left, we will do:
40 - 7. 4 = 40 - 28 = 12 m
Looking at the figure, we see that we have 6 spaces with a distance equal to d and 2 spaces with a distance equal to 2d. Thus, the sum of these distances must equal 12 m, so:
6d + 2. 2d = 12
6d + 4d = 12
10d = 12
Therefore, it is correct to say that d is equal to 1.20 m.
question 8
(CEFET/MG - 2018) In a family with 7 children, I am the youngest and 14 years younger than my mother's eldest. Among the children, the fourth is a third of the older brother's age, plus 7 years. If the sum of our three ages is 42, then my age is a number.
a) divisible by 5.
b) divisible by 3.
c) cousin.
d) par.
Correct alternative: c) cousin.
Calling the oldest child's age x, we have the following situation:
- eldest child: x
- Youngest child: x - 14
- Fourth child:
Considering that the sum of the age of the three siblings is equal to 42, we can write the following equation:
To find the age of the youngest, just do:
21 - 14 = 7 (prime number)
So if the sum of our three ages is 42, then my age is a prime number.
question 9
(EPCAR - 2018) A used car dealership presents a model and advertises it for x reais. To attract customers, the reseller offers two forms of payment:
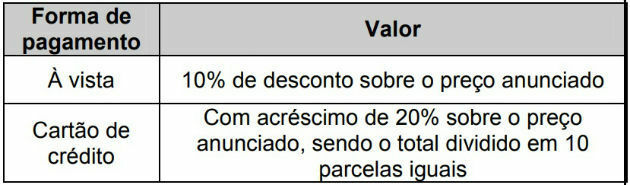
A customer purchased a car and opted to pay by credit card in 10 equal installments of R$ 3,240.00 Considering the above information, it is correct to state that
a) the value x advertised by the reseller is less than R$25,000.00.
b) if this customer had opted for cash payment, then he would have spent more than R$24,500.00 with this purchase.
c) the option that this buyer made using the credit card represented a 30% increase over the amount that would be paid in cash.
d) if the customer had paid in cash, instead of using the credit card, then he would have saved more than R$8000.00.
Correct alternative: d) if the customer had paid in cash, instead of using the credit card, then he would have saved more than R$8000.00.
Solution 1
Let's start by calculating the x value of the car. We know that the customer paid in 10 installments equal to R$3240 and that in this plan, the value of the car is increased by 20%, so:
Now that we know the value of the car, let's calculate how much the customer would pay if they opted for the cash plan:
In this way, if the customer had paid in cash, he would have saved:
32400 - 24 300 = 8 100
Solution 2
An alternative way to solve this problem would be:
1st step: determine the amount paid.
10 installments of R$ 3 240 = 10 x 3 240 = R$ 32 400
2nd step: determine the car's original value using the rule of three.
Thus, as the amount paid was increased by 20%, the original price of the car is R$ 27 000.
3rd step: determine the value of the car when making the cash payment.
27 000 - 0.1 x 27 000 = 27 000 - 2 700 = 24 300
Therefore, paying cash with a 10% discount, the final value of the car would be R$ 24,300.
Step 4: Determine the difference between cash and credit card payment terms.
R$ 32 400 - R$ 24 300 = R$ 8 100
In this way, by opting for a cash purchase, the customer would have saved more than eight thousand reais in relation to the payment in installments on the credit card.
See too: Equation Systems
question 10
(IFRS-2017) Pedro had x reais from his savings. He spent a third at the amusement park with friends. The other day, he spent 10 reais on stickers for his football players album. Then he went out to have a snack with his classmates at school, spending 4/5 more than he still had and still got a change of 12 reais. What is the value of x in reais?
a) 75
b) 80
c) 90
d) 100
e) 105
Correct alternative: e) 105.
Initially, Pedro spent of x, then spent 10 reais. In the snack he spent
of what is left after having made the previous expenses, that is,
in
, leaving 12 reais.
Considering this information, we can write the following equation:
Therefore, the value of x in reais is 105.
Keep testing your knowledge:
- Exercises on 1st Degree Equation with an Unknown
- Exercises on High School Equations
- Exercises on 1st Grade Function
- Exercises on Rule of Three
- Exercises on 1st Degree Equation Systems

