You've probably seen many fractions and decimal numbers out there, but did you know they have something in common? Fractions and decimal numbers belong to the same numeric set, O Set of Rational Numbers, which is represented by the letter 
.
But what are Rational Numbers?
In general, we say that every number written in the form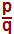 
is a rational number, where P and what are whole numbers and what ≠0. Notice that 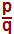can be positive or negative, since P and what are whole.
But what do decimal numbers have to do with all this?
Have you ever heard that every fraction is a division? Well then, if we have a fraction of the type 
, we can represent it as 0,5, since by dividing the numerator 1 by the denominator 2, we get the quotient 0,5. Therefore, we can say that decimals and fractions are alternatives to represent the same rational number. Let's look at some examples of integers expressed as decimals:
3 = 0,75
4
– 17 = – 8,5
2
100 = – 12,5
– 8
12 = 2,4
5
Curiosity: The letter was chosen to represent the set of rational numbers because
quotient begins with what and it is the result of a division. As already said, every fraction is a division.
And the natural numbers and are integers rational too?
Both natural numbers and whole numbers can be classified as rational numbers, as each can be expressed as a fraction. Let's look at some examples:
20 = 5
4
– 100 = – 10
10
27 = – 3
–9
10 = 2
5
We can then say that the set of numbers natural (
) it's the set ofs whole numbers (
) belong to set of rational numbers ().
Periodic tithes and generating fraction
There is a special class of rational numbers that is made up of the periodic tithes — infinite decimal numbers that are the result of inexact divisions. For example, given the fraction 
, if we divide your numerator 1 by the denominator 3, we will get the quotient 0,333333... Note that the number 3 repeats infinitely, so this quotient can be called the periodic decimal and the fraction which gave rise to it is called generating fraction.
Let's look at examples of other periodic decimals and their respective generating fractions:
15 = 1,6666...
9
– 12 = – 0,148148148...
81
7 = 0,0388888...
180
5 = – 0,185185185...
–27
Take the opportunity to check out our video lesson on the subject:
