Coordinates of the vertex of the parabola

One high school function is the one that can be written in the form f(x) = ax² + bx + c. All high school function is geometrically represented by a parable, which is a geometric figure flat. The parables connected to functions of the second degree have a maximum point or a minimum point. The biggest candidate for one of these points is called vertex of the parabola.

Getting the vertex coordinates

At vertex coordinates can be obtained in two ways. The first uses one of the following formulas:

x_v = - B
2nd

y_v = – Δ
4th

In these formulas, x_v and y_v are the coordinatesofvertex of the function of the seconddegree, that is, V(x_vy_v).

The second way to find the coordinates of the vertex is as follows: suppose x₁ and x₂ be the roots of a function of the seconddegree, the midpoint between the roots will be the x coordinate of the vertex. Knowing this, just find the image of this value through the occupation analyzed. So, given the x roots₁ and x₂ of a function f(x) = ax² + bx + c, we have:

x_v = x₁ + x₂
2

y_v = f(x_v) = ax_v² + bx_v + c

This is the second technique used to demonstrate the given formulas.

Do not stop now... There's more after the advertising ;)

Demonstration of formulas

Given a function of the second degree any f (x) = ax² + bx + c, with roots x₁ and x₂, we can find the x coordinate_v calculating the average between these roots. To do this, remember that:

x₁ = – b + √Δ
2nd 

x₂ = - B - √Δ
2nd

Therefore:

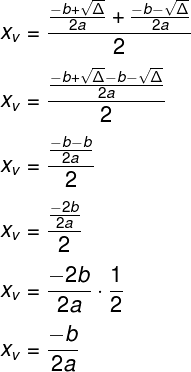
Replacing this value in the occupation f(x) = ax² + bx + c, we have:

Doing the least common multiple of the denominators, we find:

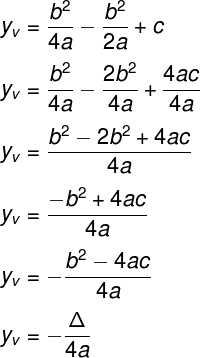
Example

Find the coordinates of the vertex of the occupation f(x) = x² – 16.

Using the formulas, we get:

x_v = - B
2nd

x_v = – 0
2

x_v = 0

y_v = – Δ
4th

y_v = - (B² – 4·a·c)
4th

y_v = – (0² – 4·1·(– 16))
4

y_v = – (– 4·(– 16))
4

y_v = – (64)
4

y_v = – 16

At coordinatesofvertex of this function are V (0, – 16).

By Luiz Paulo Moreira
Graduated in Mathematics

Would you like to reference this text in a school or academic work? Look:

SILVA, Luiz Paulo Moreira. "Coordinates of the vertex of the parabola"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/coordenadas-vertice-parabola.htm. Accessed on June 29, 2021.