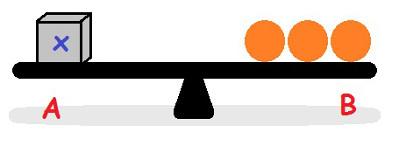
The study of equations can be daunting at first, but their development is quite simple. Let's look at a situation involving the algebraic principle of equations. In the scale above, consider that each ball has the same weight, what could we do so that both sides had the same amount of balls? We can clearly see that it is necessary to remove a ball from side A and at the same time add a ball to side B. This way, each side of the scale would have the same amount of balls and the same weight.
Let's imagine another situation: in the image below, the box has a certain weight, what should you do to find this weight?

looking for box weight
First, we must leave the name box x alone on the side THE of the scale, to do this, we must remove the two balls that are on the side THE and then add the two balls to the side B. Follow:
The box has a weight equal to the three balls
The way we move the balls made the scales balance. This indicates that the box has the same weight as the three balls. Let's see how this happens in Algebra:
x - 2 = 1
Recalling our previous example, this situation indicates the moment when the scale was not balanced. To try to balance it, we need to leave the box alone. So we'll do that here too. The action on one side of the scale is contrary to the action on the other side of the scale (Remember that we withdraw two balls on the A side and we add two balls beside B?). Therefore, we must remove this -2 on the left side and put the +2 on the right side. We will then have:
Do not stop now... There's more after the advertising ;)
x = 1 +2
x = 3
Whenever we are going to solve an equation, we need to be clear about the objective of leaving our letter (unknown, it represents the value we want to figure out) alone on one side of the equation. To do this, we need the numbers to change sides, always doing the reverse operation they are doing. It's good that we change sides first the numbers that are farthest from the unknown. Let's look at other examples:
|
5.n = 15 n = 15 n = 3 |
The = 132 a = 132. 6 a = 792 |
3.y+ 10 = 91 3.y = 91 - 10 3.y = 81 y = _81 y = 27 |
2.x + 4 = 10 2.x = 10 – 4 2.x = 6 2.x = 6. 5 2.x = 30 x = 302 x = 15 |
By Amanda Gonçalves
Graduated in Mathematics
Would you like to reference this text in a school or academic work? Look:
RIBEIRO, Amanda Gonçalves. "Introduction to the 1st Degree Equation"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/introducao-equacao-1-o-grau.htm. Accessed on June 27, 2021.
1st degree equation, Equation, Equivalent equation, Equality, Mathematical equality, Principles of equality, Additive principle of equality, Multiplication principle of equality.