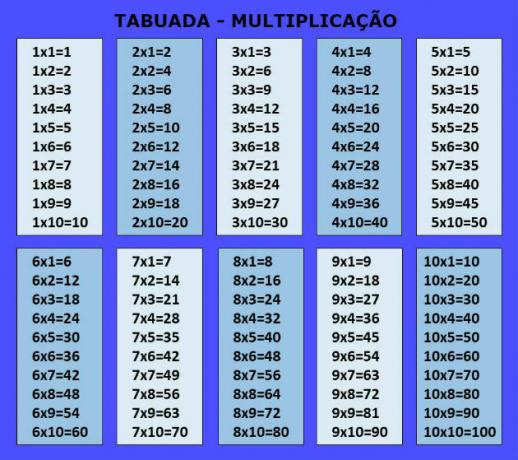
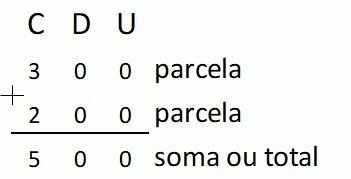
To decompose a number is to represent its digits with the place value. In numbers, each digit represents a number of units, depending on its position. By writing the sum of the units represented by each digit, we are decomposing the number.
The decomposition of the number 12 is 10 + 2, as 1 represents a ten or ten units. Likewise, the decomposition of 234 is 200 + 30 + 4, as the two represents two hundreds, the three the number of tens, and the 4 the units.
In the numbering system we use, the value of the digits depends on their position, where each one represents a certain number of units.
How to decompose a number
To decompose a number, we multiply each digit by its position value (...1000, 100, 10 ,1). Results are presented as a sum.
Thus, the 1st order digit is multiplied by 1, the tens digit by 10, the hundreds digit by 100, and so on.
Examples of decomposition
Number decomposition exercises
Exercise 1
decompose the numbers
a) 564
b) 89
c) 2034
d) 87 785
e) 201 654
a) 500 + 60 + 4
b) 80 + 9
c) 2000 + 0 + 30 + 4
d) 80 000 + 7 000 + 700 + 80 + 5
e) 200 000 + 0 + 1 000 + 600 + 50 + 4
Exercise 2
compose the numbers
a) 50 + 4
b) 600 + 30 + 8
c) 3 000 + 200 + 0 + 1
d) 40 000 + 300 + 50 + 2
e) 100 000 + 50 000 + 6 000 + 0 + 60 + 1
a) 50
b) 638
c) 3201
d) 40 352
e) 126 061
The Decimal Numbering System
Our numbering system uses ten symbols called numerals (0, 1, 2, 3, 4, 5, 6, 7, 8, 9) to write all numbers.
This is possible thanks to the system of positions with different values, where each position (order) on the left has its digit multiplied by ten, in relation to the value of the previous order.
These positions are arranged from right to left and called orders. Thus, the first order is that of units. In the second order, to the left of the first, the digit is multiplied by ten. In the third order, to the left of the second, the digit is multiplied by one hundred.
The place value of each order on the left represents 10 times the previous one, so this way of organizing and writing the numbers is called the Decimal Numbering System.
See too Decimal Numbering System.