Equations of the type ax² + bx + c = 0, where a, b and c are numerical coefficients belonging to the set of real numbers, with a ≠ 0, are called 2nd degree equations. Like all equations, they result in a solution set called the root. The difference between these equations in relation to those of the 1st degree is that they can have three different solutions according to the value of the discriminant, represented by the Greek letter ∆ (delta). Watch:
∆ > 0, the equation has two real and distinct roots.
∆ = 0, the equation has equal real roots.
∆ < 0, the equation has no real roots.
The resolution of a 2nd degree equation depends on the value of delta and a mathematical expression associated with the Indian Bhaskara. This expression consists of an efficient method of solving this equation model, based on numerical coefficients.

Example 1
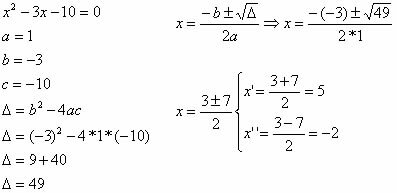
S = (x Є R / x = –2 and x = 5}
Example 2

S = (y Є R / y = 2/3}
Example 3
5x² +3x +5 = 0
a = 5
b = 3
c = 5
Δ = b² - 4ac
Δ = 3² - 4 ∙ 5 ∙ 5
Δ = 9 – 100
Δ = - 91
S = { } (there is no real solution)
by Mark Noah
Graduated in Mathematics
Source: Brazil School - https://brasilescola.uol.com.br/matematica/raiz-uma-equacao-2-grau-1.htm
