Relative Density of Gases

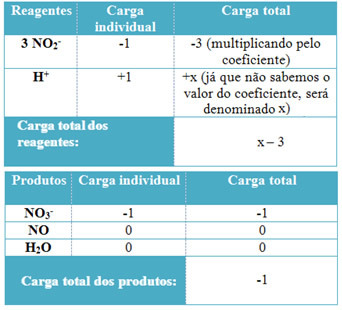
The relative density (δ) is given by the quotient between the absolute densities of two gases, under the same temperature and pressure conditions.

Let's say we have gas 1 and gas 2, so the relative density will be:

δ₁₂ = d₁
d₂

Note that relative density has no unity, as it is a pure number that only indicates how many times one gas is more or less dense than another. Since it is just a pure number, it is unaffected by variations in temperature and pressure. However, it is important that these two quantities are the same for the two gases.

We have that density is the ratio of mass to volume occupied by the gas (d = m/V). So, we can replace this relationship in the formula above:

δ₁₂ = d₁
d₂
δ₁₂ = m₁/V₁
m₂/V₂

In CNTP (Normal Conditions of Temperature and Pressure), every gas occupies a volume of 22.4 L. So, in this case, V₁ = V₂, which can be overridden in the formula above.

δ₁₂ = m₁
m₂

Relative density is proportional to the masses of gases.

Let's look at an example of how to determine relative density:

Example: What are the relative densities of two gases A and B, knowing that:

Gas A: m = 33g and V = 11 L;

Gas B: m = 24.2 g and V = 12.1 L.

Resolution:

d_THE = m = 33g = 3 g/L
V 11L

d_B = m = 24.2g = 2 g/L
V 12.1L

δ_AB = d_THE
d_B
δ_AB = 3 g/L
2 g/L
δ_AB = 1,5

We can also relate the relative density with the gas state equation (PV = nRT), as explained in the text Absolute Gas Density, We have to:

d = PM
RT

Then:

d₁ = PM₁
RT

d₂ = PM₂
RT

d₁= PM₁/RT
d₂ PM₂/RT

d₁ = M₁
d2_B M₂

Do not stop now... There's more after the advertising ;)

Note that the relative density is proportional to the molar masses of gases, this means that, in comparative terms, the greater the molar mass of a gas, the greater its density. For example, the molar mass of air is 28.96 g/mol, that of helium gas is 4 g/mol, and that of carbon dioxide is 44 g/mol.

This means that the density of helium gas in relation to air is lower. That's why when you fill a balloon with helium gas and let it go, it tends to rise. On the other hand, carbon dioxide is denser than air, so when we fill a balloon with the “air” from our lungs, we are actually filling the balloon with carbon dioxide. In this way, if we release the balloon in the air, it will tend to fall.

If a balloon containing gas with a molar mass less than 28.96 g/mol is released into the air, it will rise; but if it's bigger, it will go down

Considering air (which is a mixture of gases) as a reference, we have that the relative density of any gas in relation to it can be given by the formula:

δ_air = _M_
28,9
M = 28.9. δ_Ther

If the reference gas is another one, just substitute their respective values. For example, in the case of hydrogen gas, its molar mass is 2 g/mol, so we have:

δ_H2 = _M_
2

M = 2. δ _H2

* Editorial Credit: Keith Bell / Shutterstock.com

By Jennifer Fogaça
Graduated in Chemistry

Would you like to reference this text in a school or academic work? Look:

FOGAÇA, Jennifer Rocha Vargas. "Relative Density of Gases"; Brazil School. Available in: https://brasilescola.uol.com.br/quimica/densidade-relativa-dos-gases.htm. Accessed on June 27, 2021.

Gases

What are gases, what are the properties of gases, molecular compounds, compressibility, fixed volume, kinetic energy mean, absolute temperature of a gas, ideal gas, Real gases, perfect gas, gas state variables, volume of a gas, seasons