THE factorization it is directly related to multiplication, given that the factors are the terms that we multiply to generate the product. Look:
2 → factor 26 → factor
x 3 → factor x 7 → factor
6 → Product 182 → Product
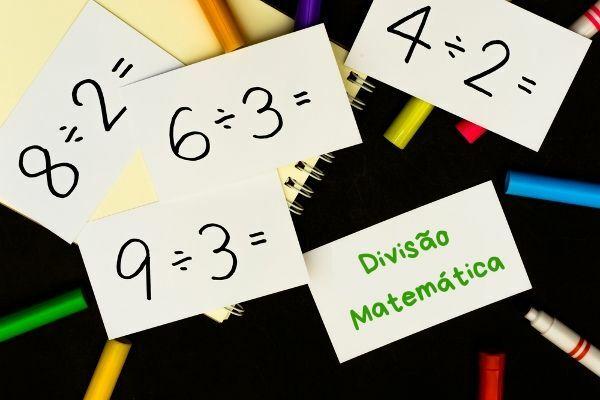
You prime factors of decomposition are obtained through successive divisions. Remember that for a number to be prime, it must be divisible only by 1 and itself, so the numbers 2, 3, 5, 7, and 11 are prime. The prime number is considered a factor when it is the divisor in the division algorithm. The structure of the division algorithm is as follows:
Dividend | Divider
Remainder Quotient
By dividing 4 by 2, we have the following situation:

Using the successive divisions, we obtain the complete factorization, which represents the decomposition of a number into prime factors. See an example of successive divisions of the number 112 and then complete factorization.
Example: Decompose the number 112 into prime factors:
112| 2
0 56 | 2
0 28 | 2
0 14 |2
0 7 |7
0 1
Every time you decompose a number into prime factors, remember that the divisor will always be a prime number and the order of succession of these divisors, which are factors, is increasing. We change the prime number of the divisor only when it is no longer possible to use it in division. In the example above, the divisor changed from number 2 to seven, since the dividend is now seven and the only divisor for 7 is 7.
Do not stop now... There's more after the advertising ;)
Still on the example above, the complete factorization of 121 is:
112 = 2. 2. 2. 2. 7 = 24. 7
In addition to the structure of the division algorithm, there is another structure that can be used to factor a number. See the following three examples:
Example: Find the complete factored form of the numbers 234, 180 and 1620:
234|2
117|3
39|3
13|13
1|
The full factored form of the number 234 is: 2. 3. 3. 13 = 2. 32. 13
Note that all factors are prime numbers and that the succession of factors takes place in an increasing way.
180|2
90|2
45|3
15|3
5|5
1|
The full factored form of the number 180 is: 2. 2. 3. 3. 5 = 22. 32. 5
All terms that make up the factorization are prime numbers.
1620|2
810|2
405|3
135|3
45|3
15|3
5|5
1|
The full factored form of the number 1620 is: 2. 2. 3. 3. 3. 3. 5 = 22. 34. 5
All numbers that make up the factorization are prime.
By Naysa Oliveira
Graduated in Mathematics
Would you like to reference this text in a school or academic work? Look:
OLIVEIRA, Naysa Crystine Nogueira. "Decomposition of a number into prime factors"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/decomposicao-um-numero-fatores-primos.htm. Accessed on June 28, 2021.