THE MondaylawinOh M describe which physical greatness relate to electrical resistance on one conductor. According to this law, the electrical resistance of a homogeneous conductor is directly proportional to its length and inversely proportional to the cross-sectional area of that conductor.
Lookalso:Learn what it is and learn how to calculate electrical current
Ohm's 2nd Law Formula
THE MondaylawinOh M shows that the resistance of a driver is related to your form but also to microscopic factors, described on the basis of a physical quantity called resistivity.
THE resistivity it is the physical magnitude that measures the opposition that some material offers to the flow of electrical charges, that is, high resistivity materials offer great resistance to the passage of electric current.
Ohm's second law formula is shown below, note:
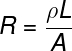
R – Resistance (Ω)
ρ – Resistivity (Ω.m)
there – Length (m)
THE – Cross-sectional area (m²)
According to this formula, the electrical resistance of a conducting wire is
directly proportional to its length, in addition, it is inversely proportional to its cross-sectional area (colloquially called the gauge). That's why we use thicker wires in applications that demand electrical currents from high intensity — they have lower electrical resistance and, therefore, dissipate less energy in form of heat.Resistivity
Ohm's second law indicates that electrical resistance is a property of the body, since this feature directly depends on spatial measurements, such as the cross-sectional area or length of the body.
THE resistivity (ρ) is a scalar physical quantity (measured in Ω.m) that does not depend on the dimensions of the body, but on microscopic properties, such as the the amountinelectrons conduction of the material and also the distance these electrons can travel without colliding with the atoms that make up the material.
See too: Find out what the elements of an electrical circuit are and how they work
Ohm's first law
According to Ohm's first law, the ratio between electric potential and electric current is always constant in ohmic resistors, as shown in the following formula:
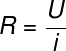
U – electrical voltage or potential difference (V)
R – electrical resistance (Ω)
i – electric current (A)
According to the formula, electrical resistance is the measure related to the current formed by applying a electric potential: the greater the electrical resistance of a material, the greater the electrical potential required for the establishment of an electrical current to occur.
Lookalso: Learn how to calculate the electromotive force of electrical generators
Solved exercises on Ohm's second law
Question 1) Determine the electrical resistance of a conductor wire 20 meters long, with a cross-sectional area of 8 mm² and resistivity equal to 1.7.10-8 Ω.m.
a) 625 Ω
b) 4.25 Ω
c) 150 Ω
d) 32 Ω
e) 25 Ω
Template: Letter B
Resolution:
Before we can calculate the electrical resistance, we need to convert the cross-sectional area of the wire, which is in mm², to the unit of m² (8 mm² = 8.10-6 m²).
To calculate the resistance of this lead wire, we will make use of Ohm's second law, note:
According to the calculation, the correct alternative is the letter b.
Question 2) It has a cylindrical conductor wire, resistance R, resistivity ρ, length L and cross-sectional area A. Keeping the other parameters fixed, what should be the electrical resistance, written in terms of R, of a wire with a diameter four times larger?
a) 8R
b) R/4
c) 2R
d) R/16
e) R/8
Template: Letter D
Resolution:
As described in the statement, the wire has a cylindrical shape, this indicates that the cross-sectional area of this wire is circular. The area of a circle, in turn, is proportional to the square of the radius (A α r²), therefore, if the second wire is four times larger in diameter, its radius will be four times larger, and its cross-sectional area will be 16 times larger.
Since the cross-sectional area of the wire is 16 times smaller, its resistance will be 16 times smaller, so the correct alternative is the letter d.
Question 3) Regarding Ohm's second law, tick the correct alternative:
a) Electrical resistance depends on both geometric and microscopic factors.
b) The electrical resistance does not depend on any macroscopic factors such as the length or cross-sectional area of the conductor.
c) The electrical resistance is a vector physical quantity measured in Ω.m.
d) The electrical resistance is directly proportional to the cross-sectional area of the wire.
e) Strength and resistivity are inversely proportional quantities.
Template: Letter a
Resolution:
Let's analyze the alternatives:
The - REAL.
B - FALSE. Electrical resistance is macroscopic and depends on geometric factors, however, it depends on resistivity, which is of microscopic origin.
ç - FALSE. Electrical resistance is scalar and its unit of measure is only Ω.
d – FALSE. Electrical resistance and cross-sectional area are inversely proportional quantities.
and - FALSE. Strength and resistivity are directly proportional physical quantities.
By Rafael Hellerbrock
Physics teacher
Source: Brazil School - https://brasilescola.uol.com.br/fisica/segunda-lei-ohm.htm
