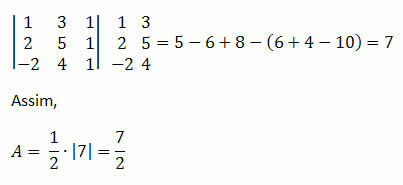We know that the value of the slope of a straight line is the tangent of its angle of inclination. Through this information we can find a practical way to obtain the value of the slope of a straight line without having to use the tangent calculation.
It is noteworthy that if the line is perpendicular to the axis of the abscissa, the angular coefficient will not exist, as it is not possible to determine the tangent of the 90º angle.
To represent a non-vertical line in a Cartesian plane, it is necessary to have at least two points belonging to it. Thus, consider a line s that passes through points A(xA, yA) and B(xB, yB) and has a slope angle with axis Ox equal to α.
Extending the ray that passes through point A and is parallel to axis Ox, we will form a right triangle at point C.
The angle A of the triangle BCA will be equal to the slope of the line, since, by Thales's Theorem, two parallel lines cut by a transversal line form equal corresponding angles.
Taking into account the triangle BCA and that the slope is equal to the slope angle tangent, we will have:
Do not stop now... There's more after the advertising ;)
tgα = opposite side / adjacent side
tgα = yB - yTHE / xB – xTHE
Therefore, the calculation of the angular coefficient of a straight line can be done by reason of the difference between two points belonging to it.
m = tgα = Δy / Δx
Example 1
What is the slope of the line that passes through points A (–1.3) and B (–2.4)?
m = Δy/Δx
m = 4 - 3 / (-2) - (-1)
m = 1 / -1
m = -1
Example 2
The angular coefficient of the straight line that passes through points A (2.6) and B (4.14) is:
m = Δy/Δx
m = 14 - 6/4 - 2
m = 8/2
m = 4
Example 3
The angular coefficient of the straight line passing through points A (8.1) and B (9.6) is:
m = Δy/Δx
m = 6 - 1/9 - 8
m = 5/1
m = 5
by Mark Noah
Graduated in Mathematics
Would you like to reference this text in a school or academic work? Look:
SILVA, Marcos Noé Pedro da. "Calculation of the slope of a straight line"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/calculo-coeficiente-angular-uma-reta.htm. Accessed on June 29, 2021.