Standard Deviation Exercises Explained

Study and answer your questions about standard deviation with the exercises answered and explained.

question 1

A school is organizing an Olympics where one of the tests is a race. The times it took five students to complete the test, in seconds, were:

23, 25, 28, 31, 32, 35

The standard deviation of the students' test times was:

See Answer

Answer: Approximately 3.91.

The standard deviation can be calculated by the formula:

$DP equals square root of numerator start style show sum of straight i equals 1 to straight n end of style parenthesis left straight x with straight i subscript minus MA right parenthesis squared over straight denominator n end of fraction end of source$

Being,

∑: summation symbol. Indicates that we have to add all the terms, from the first position (i=1) to the n position
x_i: value at position i in the dataset
M_A: arithmetic mean of the data
n: amount of data

Let's solve each step of the formula separately, to make it easier to understand.

To calculate the standard deviation, it is necessary to calculate the arithmetic mean.

$MA equals numerator 23 space plus space 25 space plus space 28 space plus space 31 space plus space 32 space plus space 35 over denominator 6 end of fraction equals 174 over 6 equals 29$

We now add the subtraction of each term by the mean squared.

left parenthesis 23 space minus space 29 right parenthesis squared plus left parenthesis 25 minus 29 right parenthesis squared plus left parenthesis 28 minus 29 right parenthesis squared plus left parenthesis 31 minus 29 right parenthesis squared plus left parenthesis 32 minus 29 right parenthesis squared plus parenthesis left parenthesis 35 minus 29 right parenthesis squared equals space left parenthesis minus 6 right parenthesis squared plus left parenthesis minus 4 right parenthesis squared squared plus left parenthesis minus 1 right parenthesis squared plus 2 squared plus 3 squared plus 6 squared equals 36 plus 16 plus 1 plus 4 plus 9 plus 36 equal to 92

We divide the value of this sum by the number of elements added.

92 over 6 approximately equals 15 point 33

Finally, we take the square root of this value.

square root of 15 point 33 end of root approximately equal 3 point 91

question 2

The same assessment was applied to four groups with different numbers of people. The minimum and maximum scores for each group are shown in the table.

instagram story viewer

Considering the average of each group as the arithmetic mean between the minimum and maximum grade, determine the standard deviation of the grades in relation to the groups.

Consider up to the second decimal place, to simplify the calculations.

See Answer

Answer: approximately 1.03.

The standard deviation can be calculated by the formula:

$DP equals square root of numerator start style show sum of straight i equals 1 to straight n left square bracket x with straight i subscript minus MA right square parenthesis end of style over straight denominator n end of fraction end of source$

As the quantities are different in each group, we calculate the arithmetic mean of each one, then weight it between the groups.

Arithmetic averages

A colon space left parenthesis 89 minus 74 right parenthesis divided by 2 equals 7 comma 5 B colon space left parenthesis 85 minus 67 right parenthesis divided by 2 equals 9 C colon space left parenthesis 90 minus 70 right parenthesis divided by 2 equals 10 D colon space left parenthesis 88 minus 68 right parenthesis divided by 2 equal to 10

Weighted average between groups

$M P equals space numerator 7 comma 5 space. space 8 space more space 9 space. space 12 space more space 10 space. space 10 space more space 10 space. space 14 over denominator 8 plus 12 plus 10 plus 14 end of fraction M P equals numerator 60 plus 108 plus 100 plus 140 over denominator 44 end of fraction M P equals 408 over 44 approximately equals 9 point 27$

Term calculation:

sum of straight i equals 1 to straight n left parenthesis straight x with straight i subscript minus M P right squared parenthesis , where xi is the mean of each group.

left parenthesis 7 comma 5 minus 9 comma 27 right parenthesis squared plus left parenthesis 9 minus 9 comma 27 right parenthesis squared plus parenthesis left 10 minus 9 comma 27 right parenthesis squared plus left parenthesis 10 minus 9 comma 27 right parenthesis squared equals space open parentheses minus 1 comma 77 close squared parenthesis plus left parenthesis minus 0 comma 27 right squared parenthesis plus left parenthesis 0 comma 73 right parenthesis square plus left parenthesis 0 comma 73 right parenthesis squared equals space 3 comma 13 plus 0 comma 07 plus 0 comma 53 plus 0 comma 53 equals 4 comma 26

Dividing the sum value by the number of groups:

$numerator 4 comma 26 over denominator 4 end of fraction equal to 1 comma 06$

Taking the square root

square root of 1 point 06 end of root approximately equal 1 point 03

question 3

In order to implement quality control, an industry that produces padlocks monitored its daily production for a week. They recorded the number of defective padlocks produced each day. The data were as follows:

Monday: 5 defective parts
Tuesday: 8 defective parts
Wednesday: 6 defective parts
Thursday: 7 defective parts
Friday: 4 defective parts

Calculate the standard deviation of the number of defective parts produced during that week.

Consider up to the second decimal place.

See Answer

Answer: Approximately 1.41.

To calculate the standard deviation, we will calculate the average between the values.

$MA equals numerator 5 plus 8 plus 6 plus 7 plus 4 over denominator 5 end of fraction equals 30 over 5 equals 6$

Using the standard deviation formula:

$DP equals square root of numerator start style show sum of square i equals 1 to square n of left square bracket x with square i subscript minus MA right square squared end of style over straight denominator n end of fraction end of DP root equals square root of numerator start style show left parenthesis 5 minus 6 right squared parenthesis plus left parenthesis 8 minus 6 right parenthesis squared plus left parenthesis 6 minus 6 right parenthesis squared plus left parenthesis 7 minus 6 right parenthesis square plus left parenthesis 4 minus 6 right parenthesis squared end of style over denominator 5 end of fraction end of root DP equals square root of numerator start style show left parenthesis minus 1 right parenthesis squared plus 2 squared plus 0 squared plus 1 squared plus left parenthesis minus 2 right parenthesis squared end style over denominator 5 end of fraction end root DP equals square root of numerator start style show 1 plus 4 plus 0 plus 1 plus 4 end style over denominator 5 end of fraction end of root DP equals square root of numerator start style show 10 end of style over denominator 5 end of fraction end of root equals square root of 2 approximately equals 1 point 41$

question 4

A toy store surveyed the company's revenue over the course of a year and obtained the following data. in thousands of reais.

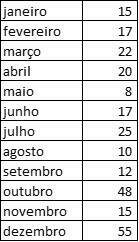
Table with data associated with the question.

Determine the standard deviation of the company's revenue this year.

See Answer

Answer: approximately 14.04.

Calculating the arithmetic mean:

$MA equals numerator 15 plus 17 plus 22 plus 20 plus 8 plus 17 plus 25 plus 10 plus 12 plus 48 plus 15 plus 55 over denominator 12 end of fraction MA equals 264 over 12 equals 22$

Using the standard deviation formula:

To calculate the sum:

left parenthesis 15 minus 22 right parenthesis squared equals 49 left parenthesis 17 minus 22 right parenthesis squared equals 25 left parenthesis 22 minus 22 right parenthesis squared equals 0 left parenthesis 20 minus 22 right parenthesis squared equals 4 left parenthesis 8 minus 22 right parenthesis squared equals 196 left parenthesis 17 minus 22 right parenthesis squared equals 25 left parenthesis 25 minus 22 right parenthesis squared equals 9 left parenthesis 10 minus 22 right parenthesis squared equals 144 left parenthesis 12 minus 22 right parenthesis squared equals 100 left parenthesis 48 minus 22 parenthesis right squared equals 676 left bracket 15 minus 22 right bracket squared equals 49 left bracket 55 minus 22 right bracket squared equals 1089

Adding all the installments we have 2366.

Using the standard deviation formula:

$DP equals square root of numerator start style show 2366 end style over denominator 12 end of fraction end root approximately equal square root of 197 point 16 end root approximately equal 14 comma 04$

question 5

Research is being carried out with the aim of knowing the best variety of a plant for agricultural production. Five samples of each variety were planted under the same conditions. The regularity in its developments is an important feature for large-scale production.

Their heights after a certain time are below, and the plant variety with greater regularity will be chosen for production.

Variety A:

Plant 1: 50 cm
Plant 2: 48 cm
Plant 3: 52 cm
Plant 4: 51 cm
Plant 5: 49 cm

Variety B:

Plant 1: 57 cm
Plant 2: 55 cm
Plant 3: 59 cm
Plant 4: 58 cm
Plant 5: 56 cm

Is it possible to arrive at a choice by calculating the standard deviation?

See Answer

Answer: It is not possible, as both varieties have the same standard deviation.

Arithmetic mean of A

$MA equals numerator 50 plus 48 plus 52 plus 51 plus 49 over denominator 5 end of fraction equals 250 over 5 equals 50$

standard deviation of A

$DP equals square root of numerator start style show sum of square i equals 1 to square n of left square bracket x with square i subscript minus MA right square squared end of style over straight denominator n end of fraction end of DP root equals square root of numerator start style show left parenthesis 50 minus 50 right squared parenthesis plus left parenthesis 48 minus 50 right parenthesis squared plus left parenthesis 52 minus 50 right parenthesis squared plus left parenthesis 51 minus 50 right parenthesis square plus left parenthesis 49 minus 50 right parenthesis squared end of style over denominator 5 end of fraction end root DP equals square root of numerator start style show 0 squared plus left parenthesis minus 2 right parenthesis squared plus 2 squared plus 1 squared plus left parenthesis minus 1 right parenthesis squared end of style over denominator 5 end of fraction end root DP equals square root of numerator start style show 0 plus 4 plus 4 plus 1 plus 1 end style over denominator 5 end of fraction end of root DP equals square root of numerator start style show 10 end of style over denominator 5 end of fraction end of root equals square root of 2 approximately equals 1 point 41$

Arithmetic mean of B

$M A equals numerator 57 plus 55 plus 59 plus 58 plus 56 over denominator 5 end of fraction equals 285 over 5 equals 57$

standard deviation of B

$DP equals square root of numerator start style show sum of straight i equals 1 to straight n left parenthesis square x with square i subscript minus MA right parenthesis to square root end of style over straight denominator n end of fraction end root DP equals square root of numerator start style show left parenthesis 57 minus 57 right parenthesis squared plus left parenthesis 55 minus 57 right parenthesis squared plus left parenthesis 59 minus 57 right parenthesis squared plus left parenthesis 58 minus 57 right squared parenthesis plus left parenthesis 56 minus 57 right squared parenthesis end of style over denominator 5 end of fraction end of root DP equals square root of numerator start style show 0 plus opening parenthesis minus 2 closing parenthesis squared plus 2 squared plus 1 squared plus left parenthesis minus 1 right parenthesis next square end of style over denominator 5 end of fraction end root DP equals square root of numerator start style show 0 plus 4 plus 4 plus 1 plus 1 end of style over denominator 5 end of fraction end of root DP equals square root of numerator start style show 10 end of style over denominator 5 end of fraction end of root equals square root of 2 equals 1 comma 41$

question 6

In a certain audition for a role in a play, two candidates entered and were evaluated by four judges, each of whom provided the following marks:

Candidate A: 87, 69, 73, 89
Candidate B: 87, 89, 92, 78

Determine the candidate with the highest mean and lowest standard deviation.

See Answer

Answer: Candidate B had the highest mean and lowest standard deviation.

Candidate A average

$MA equals numerator 87 plus 69 plus 73 plus 89 over denominator 4 end of fraction MA equals 318 over 4 MA equals 79 comma 5$

Candidate B average

$MB equals numerator 87 plus 89 plus 92 plus 78 over denominator 4 end of fraction MB equals 346 over 4 MB equals 86 comma 5$

standard deviation of A

$DP equals square root of numerator start style show sum of square i equals 1 to square n of left square bracket x with square i subscript minus MA right square squared end of style over straight denominator n end of fraction end of DP root equals square root of numerator start style show left parenthesis 87 minus 79 comma 5 right parenthesis to square plus left parenthesis 69 minus 89 right parenthesis squared plus left parenthesis 73 minus 92 right parenthesis squared plus left parenthesis 89 minus 75 parenthesis right squared end of style over denominator 4 end of fraction end of root DP equals square root of numerator 56 comma 25 plus 400 plus 361 plus 196 over denominator 4 end of fraction end of root DP equals square root of numerator 1013 comma 25 over denominator 4 end of fraction end of root DP equals square root of 506 comma 62 end of root DP equals 22 comma 5$

standard deviation of B

$DP equals square root of numerator start style show sum of square i equals 1 to square n of left square bracket x with square i subscript minus MB square right square end style over straight denominator n end of fraction end root DP equals square root of numerator start style show left parenthesis 87 minus 86 comma 5 right parenthesis to square plus opening parentheses 89 minus 86 comma 5 closing squared parenthesis plus opening parenthesis 92 minus 86 comma 5 closing squared parenthesis plus opening parenthesis 78 minus 86 comma 5 close squared parentheses end of style over denominator 4 end of fraction end of root DP equals square root of numerator 0 comma 25 plus 6 comma 25 plus 30 comma 25 plus 72 comma 25 over denominator 4 end of fraction end of DP root equal to square root of 109 over 4 end of DP root equal to square root of 27 comma 25 end of DP root approximately equal 5 point 22$

question 7

(UFBA) During a working day, a pediatrician assisted, in his office, five children with symptoms compatible with the flu. At the end of the day, he produced a table with the number of days that each of the children had a fever, before the appointment

Based on these data, it can be stated:

The standard deviation for the number of fever days for these children was greater than two.

Right

Wrong

Answer explained

Calculation of the arithmetic mean.

$MA equals numerator 3 plus 3 plus 3 plus 1 plus 5 over denominator 5 end of fraction equals 15 over 5 equals 3$

Standard deviation

$DP equals square root of numerator start style show sum of square i equals 1 to square n left parenthesis square x with square i subscript minus MA parenthesis right squared end of style over straight denominator n end of fraction end of rootDP equals square root of numerator start style show left parenthesis 3 minus 3 right parenthesis squared plus left parenthesis 3 minus 3 right parenthesis squared plus left parenthesis 3 minus 3 right parenthesis squared plus parenthesis left 1 minus 3 right squared parenthesis plus left parenthesis 5 minus 3 right squared parenthesis end of style over denominator 5 end of fraction end of rootDP equals square root of numerator start style show 0 plus 0 plus 0 plus 4 plus 4 end style over denominator 5 end fraction end rootDP equals square root of numerator start style show 8 end style over denominator 5 end fraction end root equal square root of 1 comma 6 end root space approximately equal 1 comma 26$

question 8

(UNB)

The graph above shows the number of hospitalizations of drug users up to 19 years old, in Brazil, from 2001 to 2007. The average number of hospitalizations in the period, indicated by the bold line, was equal to 6,167.

Check the option that presents the expression that allows you to correctly determine the standard deviation — R — of the data series indicated in the graph.

The) 7 straight R squared space equals space 345 squared space plus space 467 squared space plus space 419 to the power of 2 space end from exponential plus space 275 squared space plus space 356 squared space plus space 74 squared space plus space 164 squared square

B) 7 straight R space equals space √ 345 space plus space √ 467 space plus space √ 419 space plus space √ 275 space plus space √ 356 space plus space √ 74 space plus space √ 164

w) space 6,167 R squared equals 5,822 squared space plus space 6,634 squared space plus space 6,586 squared space plus space 5,892 squared space plus space 5,811 squared plus space 6,093 squared space plus space 6,331 squared square

d) 6,167 straight R equals √ 5,822 plus √ 6,634 plus √ 6,586 plus √ 5,892 plus √ 5,811 plus √ 6,093 plus √ 6,331

Answer explained

Calling the standard deviation R:

$straight R equals square root of numerator start style show sum of straight i equals 1 to straight n of left parenthesis straight x with straight i subscript minus MA right square parenthesis end of style over denominator straight n end of fraction end of source$

Squaring the two terms:

$straight R squared equals open parentheses square root of numerator start style show sum of straight i equals 1 to straight n left parenthesis straight x with straight i subscript minus MA right square parenthesis end of style over straight denominator n end of fraction end of root close square square parentheses R squared equals numerator start style show sum of square i equals 1 to square n of left parenthesis square x with square i subscript minus MA right square bracket end of style over denominator square n end of fraction$

Being n equal to 7, it passes to the left by multiplying R².

sum of straight i equals 1 to straight n of left parenthesis straight x with straight i subscript minus MA right square squared

Thus, we see that the only possible alternative is the letter a, as it is the only one in which the R appears raised to the square.

question 9

(Enem 2019) An inspector from a certain bus company records the time, in minutes, that a novice driver spends to complete a certain route. Table 1 shows the time spent by the driver on the same journey seven times. Chart 2 presents a classification for the variability over time, according to the standard deviation value.

Based on the information presented in the tables, the time variability is

a) extremely low.

b) low.

c) moderate.

d) high.

e) extremely high.

Answer explained

To calculate the standard deviation we need to calculate the arithmetic mean.

$MA equals numerator 48 plus 54 plus 50 plus 46 plus 44 plus 52 plus 49 over denominator 7 end of fraction MA equals 343 over 7 equals 49$

Standard deviation calculation

$DP equals square root of numerator start style show sum of straight i equals 1 to straight n left parenthesis square x with square i subscript minus MA right parenthesis to square root end of style over straight denominator n end of fraction end rootDP equals square root of numerator start style show left parenthesis 48 minus 49 parenthesis right squared plus left parenthesis 54 minus 49 right squared plus left parenthesis 50 minus 49 right squared plus left parenthesis 46 minus 49 right parenthesis squared plus left parenthesis 44 minus 49 right parenthesis squared plus left parenthesis 52 minus 49 right parenthesis squared plus left parenthesis 49 minus 49 right parenthesis squared end of style over denominator 7 end of fraction end of rootDP equals square root of numerator 1 plus 25 plus 1 plus 9 plus 25 plus 9 plus 0 over denominator 7 end of fraction end rootDP equals square root of 70 over 7 end of root equals square root of 10 approximately equals 3 point 16$

As 2 ＜= 3.16 ＜ 4, the variability is low.

question 10

(Enem 2021) A zootechnician intends to test whether a new rabbit feed is more efficient than the one he is currently using. The current feed provides an average mass of 10 kg per rabbit, with a standard deviation of 1 kg, fed this feed over a period of three months.

The zootechnician selected a sample of rabbits and fed them the new feed for the same period of time. At the end, he wrote down the mass of each rabbit, obtaining a standard deviation of 1.5 kg for the distribution of the masses of the rabbits in this sample.

To assess the efficiency of this ration, he will use the coefficient of variation (CV) which is a measure of dispersion defined by CV = $straight numerator S over straight denominator X in upper frame end of fraction$ , where s represents the standard deviation and straight X in top frame , the average mass of the rabbits that were fed a given feed.

The zootechnician will replace the feed he has been using for the new one, if the coefficient of variation of the mass distribution of the rabbits that were fed the new feed is less than the coefficient of variation of the mass distribution of the rabbits that were fed the feed current.

The replacement of the ration will occur if the mean of the mass distribution of the rabbits in the sample, in kilograms, is greater than

a) 5.0

b) 9.5

c) 10.0

d) 10.5

e) 15.0

Answer explained

current ration

Average mass of 10 kg per rabbit ()
1kg standard deviation

new feed

unknown mean mass
Standard deviation of 1.5 kg

condition for replacement

$CV with new subscript less than CV with current subscript straight numerator S over straight denominator X in upper frame end of fraction smaller than straight numerator S over straight denominator X in upper frame end of fraction numerator 1 comma 5 over straight denominator X end of fraction less than 1 over 1015 less than straight X$

learn more about standard deviation.

See too:

Variance and standard deviation
Statistics - Exercises
Mean, Mode and Median Exercises

ASTH, Rafael. Standard Deviation Exercises.All Matter, [n.d.]. Available in: https://www.todamateria.com.br/exercicios-de-desvio-padrao/. Access at:

See too