Cube Area Calculation: formulas and exercises

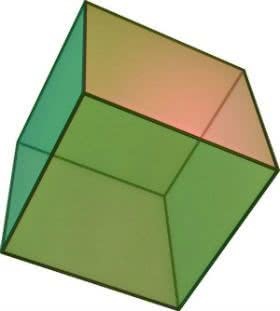
THE cube area corresponds to the measure of the surface of this spatial geometric figure.

Remember that the cube is a polyhedron, more precisely a regular hexahedron. That's because it has 6 square faces.

It is also considered a square-based prism or a rectangular parallelepiped.

All faces and edges of this figure are congruent and perpendicular. The cube has 12 edges (line segments) and 8 vertices (points).

Formulas: How to Calculate?

In relation to the area of ​​the cube, it is possible to calculate the total area, a base area and the side area.

Total area

THE total area (THE_t) corresponds to the sum of the areas of the polygons that form the figure, that is, it is the sum of the areas of the bases and the lateral area.

To calculate the total area of ​​the cube, use the following formula:

THE_t = 6th²

Where,

THE_t: total area
The: edge measurement

Base Area

THE base area (THE_B) is related to the two congruent square bases it has.

To calculate the base area, use the following formula:

THE_B = the²

Where,

THE_B: base area
The: edge measurement

Side Area

THE side area (THE_there) corresponds to the sum of the areas of the four squares that form this regular polyhedron.

To calculate the lateral area of ​​the cube, use the following formula:

THE_there = 4th²

Where,

THE_there: side area
The: edge measurement

Note: the edges of the cube are also called sides. The diagonals of this figure are straight lines between two vertices, being calculated by the formula: d = a√3.

Solved Exercises

A cube has sides measuring 5 cm. Calculate:

The) side area

See Answer

THE_there = 4.a²
THE_there = 4.(5)²
THE_there = 4.25
THE_there = 100 cm²

B) base area

See Answer

THE_B = the²
THE_B = 5²
THE_B = 25 cm²

ç) total area

See Answer

THE_t = 6.a²
THE_t = 6.(5)²
THE_t = 6.25
THE_t = 150 cm²

Entrance Exam Exercises with Feedback

1. (Fuvest-SP) Two cube-shaped aluminum blocks, with edges measuring 10 cm and 6 cm, are carried together with the fusion and then the liquid aluminum is molded as an 8 cm, 8 cm straight-edged parallelepiped cm and x cm. The value of x é:

a) 16 m
b) 17 m
c) 18 m
d) 19 m
e) 20 m

See Answer

Alternative d: 19 m

2. (Vunesp) The diagonal of the cube whose total area is 150 m², measures in m:

a) 5√2
b) 5√3
c) 6√2
d) 6√3
e) 7√2

See Answer

Alternative b: 5√3

3. (UFOP-MG) The total area of ​​a cube whose diagonal measures 5√3 cm is:

a) 140 cm²
b) 150 cm²
c) 120√2 cm²
d) 100√3 cm²
e) 450 cm²

See Answer

Alternative b: 150 cm²

Read too:

• Cube
• Cube Volume
• Polyhedron
• Prism
• Spatial Geometry