Cube Volume Calculation: formula and exercises

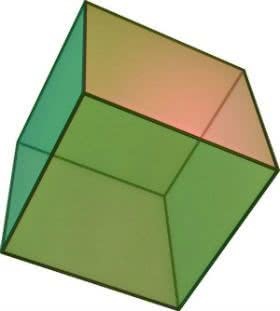
O cube volume corresponds to the space that this spatial geometric figure occupies.

Remember that the cube is a regular hexahedron, where all sides are congruent.

In terms of composition, it consists of 6 quadrangular faces, 12 edges (or sides) and 8 vertices (points).

Formula: How to Calculate?

To calculate the cube's volume, just multiply its edges three times.

This is because they are related to the length, width and depth (or height) of the figure:

V = a. The. The
or
V = a³

Where:

V: cube volume
The: edge of the cube

Solved Exercises

Calculate the volumes of the following cubes:

The) 10 m deep

See Answer

V = a³
V = (10)³
V = 1000 m³

B) 15 cm wide

See Answer

V = a³
V = (15)³
V = 3375 cm³

ç) 1.5 m long

See Answer

V = a³
V = (1.5)³
V = 3.375 m³

Generally, cube volume is indicated in cubic meters (m³) or cubic centimeters (cm³)

Did you know?

The cube is one of Plato's five Solids, alongside the tetrahedron, octahedron, dodecahedron and icosahedron.

It is also considered a square base prism or a rectangular parallelepiped.

Entrance Exam Exercises with Feedback

1. (FEI–SP) The measurements of the edges of a rectangular parallelepiped are proportional to 2, 3 and 4. If its diagonal measures 2√29 cm, its volume, in cubic centimeters, is:

a) 24
b) 24√29
c) 116
d) 164
e) 192

See Answer

Alternative e: 192

2. (Enem–2010) A factory produces chocolate bars in the shape of cobblestones and cubes, with the same volume. The edges of the parallelepiped-shaped chocolate bar measure 3 cm in width, 18 cm in length and 4 cm in thickness.

Analyzing the characteristics of the described geometric figures, the measure of the edges of the cube-shaped chocolates is equal to

a) 5 cm.
b) 6 cm.
c) 12 cm.
d) 24 cm.
e) 25 cm

See Answer

Alternative B: 6 cm.

3. (Enem-2009) A company that manufactures steel sphereş, with a radius of 6 cm, uses wooden boxes, in the shape of a cube, to transport them. Knowing that the capacity of the box is 13,824 cm³, so the maximum number of spheres that can be transported in a box is equal to

a) 4.
b) 8.
c) 16.
d) 24.
e) 32.

See Answer

Alternative b: 8.

Read too:

• Cube
• Cube Area
• Polyhedron
• Prism
• Paving stone
• Spatial Geometry