O decimal numbering system uses the number 10 as a base, in it the numbers 0, 1, 2, 3, 4, 5, 6, 7, 8 and 9 are used to count units, tens and hundreds, and so on. In this system, when we place the number 0 to the right of a digit, it is the same as multiply it by the base, that is, by 10.
Read too: Differences between number, numeral and digit
Decimal System Features
In the decimal numbering system, numbers are arranged based on the grouping of Indo-Arabic numerals, and with them it is possible to write any number.
Indo-Arabic numerals → 0, 1, 2, 3, 4, 5, 6, 7, 8, 9
Each of them represents a certain amount of unit, see:

Note that continuing to design units is laborious, so let's better understand what units are, tens, hundreds, thousands units, and so on.
One of the main aspects of this system is that: out of every 10 units, we form 1 ten (10); out of every 10 tens, we form 1 hundred (100); and out of every 10 hundreds, we form 1 unit of a thousand (1,000), that is, every time the digit 0 is added, we must multiply the order by 10.
10 units → 1 ten
10 tens → 1 hundred
10 hundreds → 1 unit of thousands
10 thousand units → 1 hundred thousand
Example 1
Determine the number of units, tens, hundreds, and so on, of the following numbers.
The) 873
Decomposing the number, we have:
873 → 800 + 70 + 3
8 hundreds (8 x 100): 800 units
7 tens (7 x10): 70 units
3 units
B) 1.327
1.327 → 1000 + 300 + 20 + 7
1 unit of thousand: 1000 units
3 hundreds (3 x100): 300 units
2 dozens (2 x 10): 20 units
7 units
See too: Ordinal numbers - nomenclature and representation
Order and class of the decimal system
Each of the digits represents an order, and we should always start by parsing them from left to right. See the table:
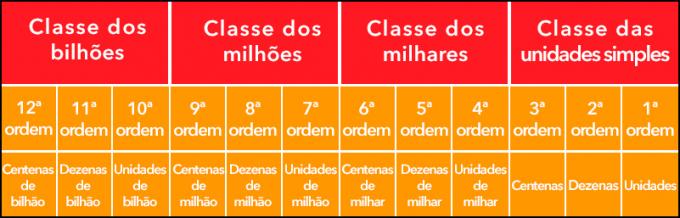
The class of a number is determined by separating it by three digits:
- Single unit class: from 1st order to 3rd order
- Thousands Class: from 4th order to 6th order
- Million Class: from the 7th order to the 9th order
- Hundreds of millions class: from the 10th order to the 12th order
Understanding the order and class of a number helps us better understand the number being worked on, for example:
a) 23431
Let's separate the number 23431 every three orders, like this:
23.431
Note that the 431 is in the single unit class, so it will read as: four hundred and thirty-one. The number 23 belongs to the thousand units class, so it will be read as: twenty-three thousand.
Therefore, the number 23,431 is read as: twenty-three thousand four hundred and thirty-one.

solved exercises
question 1 – Write the number in full and break it down. For this, indicate its order and class.
a) 1543567
Let's initially separate the orders of this number by three digits.
1.543.567
Number 567 belongs to the class of simple units; the number 543 belongs to the thousands class; and number 1 belongs to the class of millions. So let's read the number as:
One million five hundred and forty three thousand and five hundred and sixty seven
Decomposing this number, we have:
1.000.000 + 500.000 + 40.000 + 3.000 + 500 + 60 + 7
7 units
6 dozens
5 hundreds
3 thousand units
4 tens of thousands
5 hundred thousand
1 unit of a million