Internal side angles and external are observed in the encounter of a straight cross with two parallel lines. The words "collateral", "internal" and "external" refer to the positions occupied by these angles in relation to the straightcross and the straightparallel.
Remember that two lines are called parallel when there is no meeting point between them along their entire length and that the lines are infinite for two directions.
Inner and outer region
given two straightparallel, two regions can be observed: a internal and the external. The inner region is the space between the two lines, and any angle there, within the theme "inner and outer side angles", is called inner angle.
The following figure represents the space between two straightparallel.

already the regionexternal is the set of points outside two straightparallel, that is, it is the region that is not internal. The following figure illustrates the outer region of two parallel lines.

angles at regionexternal, also within this theme, are called anglesexternal.
cross straight
THE straightcross has a property: if a line t cuts a line r, which in turn is parallel to a line s, then the line t also cuts the line s.
Assuming that the straight be cross to the lines r and s, which are parallel, we will always be able to observe the formation of eight angles, four of them in the regioninternal – that's why they are called anglesinternal – and four of them in the outer region – that's why they are called anglesexternal.
Inner and Outer Side Angles
Of the eight angles formed in the two straightparallelcut off for one cross, watch the four anglesinternal: two of them are on the right side and the other two are on the left side of the transverse straight. These two angles in the internal region of two parallel lines, which at the same time are on the same side with respect to the transversal line, are called internal collaterals.
Note that the word “collateral” is used precisely because the angles are on the same side. Also, the word "internal" is used because the angles meet in the regioninternal of the two straightparallel.

Example of internal side angles
The same goes for anglescollateralexternal, with the difference that the angles are in the external region of the straightparallel.
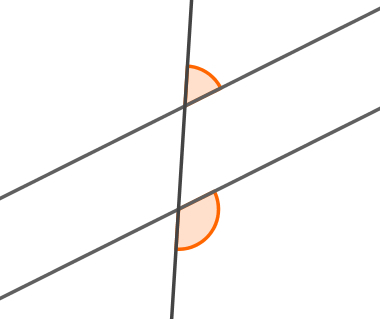
Example of external side angles
Property
two angles that are collateralinternal and two angles that are collateralexternos have the same property:
Internal side angles are supplemental.
In other words, the sum between two angles that are collateralinternal always results in 180°, just like the sum of two angles that are collateralexternal results to that same extent.
Related video lessons:



