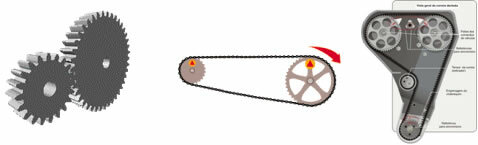
THE reason between two numbers is given by your division obeying the order in which they were given. Such ratio can be represented in fractional, decimal and percentage. The relationship between two or more reasons is an important tool to solve practical problems, this equality is called proportion.
Read too: Proportion properties: what are they and what are they for?
ratio and proportion
→ Definition of reason: consider two rational numbers x and y, with y nonzero. The ratio of x to y, in that order, is given by the quotient:

Example
The ratio between the numbers:
a) 3 and 4
b) 5 and 7
We must be very attentive to the order in which the numbers are given, the first number will always be the numerator, and the second number will always be the denominator. Look:

→ Definition of proportion: When we match two ratios, we are forming a proportion. Consider two reasons where b ≠ 0 and y ≠ 0:

Equality will be a proportion if a · y = b · x, that is, if multiplying crossed we find a true equality, then we have a proportion
Example
Check if the numbers 2, 3, 10 and 15 are proportional in that order.
For this, we must assemble the ratio between these numbers and then multiply crossed. If we find a true equality then they will be proportional, otherwise they will not be proportional.

See too: Proportionality between quantities: types and examples
Do not stop now... There's more after the advertising ;)
How to represent a reason?
We saw that a reason is given by a division, which, in turn, can be represented by one fraction. By dividing the numerator by the denominator of this fraction, we will obtain the decimal form of reason. Based on the decimal form, we can write the ratio in its percentage form, just multiplying this decimal number by 100. See the examples.
Example
Representation of the ratio between 2 and 4 in fractional, decimal and percentage form.
The ratio between 2 and 4 is given by:

To determine the decimal form, just divide the numerator by the denominator.
2 ÷ 4 = 0,5
Therefore, 0.5 is the decimal representation of the ratio of the numbers 2 and 4.
To write this ratio in percentage form, we must multiply the number 0.5 by 100. Look:
0,5 · 100 = 50%
Therefore:


solved exercises
question 1 – (Unisinos-RS) Knowing that the distance between two cities on a map, in scale 1:1600,000, is 8 cm, what is the real distance between them?
a) 2 km
b) 12.8 km
c) 20 km
d) 128 km
e) 200 km
Solution
Alternative d. From the statement we have the scale 1: 1 600 000, that is, each 1 centimeter on the map corresponds to 1 600 000 centimeters in reality. Interpreting this scale as the ratio between 1 and 1 600 000, we must determine the real average of a distance of 8 centimeters on the map, therefore:

Note that alternatives are given using the kilometer unit of measure. To turn centimeter into kilometer, we must divide the last result by 100,000:
12,800,000 ÷ 100,000 = 128 km
question 2 – The age ratio of two people is 12 to 11. It is known that the sum of the ages is 115, determine the age of each of these people.
Solution
Since we don't know the age of the two people, let's name them a and b. As the ratio between these ages is 12 to 11, we can build a ratio:

We know that the sum of ages is 115, so:
a + b = 115
a = 115 - b
Substituting the value of a in the first equation, we have:
11 · a = 12 · b
11 · (115 – b) = 12 · b
1,265 - 11b = 12b
1,265 = 12b + 11b
1,265 = 23b
b = 1,265 ÷ 23
b = 55
As a = 115 - b, then:
a = 115 - 55
a = 60
Therefore, these people are, respectively, 60 years old and 55 years old.
by Robson Luiz
Maths teacher
Would you like to reference this text in a school or academic work? Look:
LUIZ, Robson. "Reason"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/razao.htm. Accessed on June 27, 2021.