The numbers we use on a daily basis are made up of digits. These figures have an absolute value and a relative value, also called positional value.

To represent the value of a digit, we must use the Indo-Arabic numerals (1, 2, 3, 4, 5, 6, 7, 8, 9). The position in which one of these digits is found in the number can provide us with a lot of information about it. Still, we must analyze, under two aspects, this position, they are: absolute value and relative value.

Read too: Decimal numbering system - base 10 system

Absolute value of a digit

The absolute value of a digit is not associated with the position in which it is found in the number, that is, it does not depend on the position it occupies, as it represents your own quantity. See the examples:

Example 1 – In the number 1563, the digit 5 ​​has an absolute value 5 as the digit 6 has an absolute value 6.

Example 2 – In number 84, digit 8 has absolute value 8 and digit 4 has absolute value 4.

Note that analyzing the absolute value of a digit is equivalent to look in isolation for him, that is, we take neither class nor order into account.

Relative value of a digit

With these digits we can represent any numerical quantity.

The relative value of a digit it will depend on the position he is in, that is, of your order. For this reason, relative value is also called positional value.

See the examples:

Example 3 – In the number 1563, note that the digit 5 ​​is third order, that is, the order of hundreds, so the relative or positional value of the digit is 500. The number 6, on the other hand, occupies the order of tens and, therefore, its relative value is 60.

Example 4 – Determine the relative value of the digits of the number 1,563,124.

A more efficient way to determine the relative value of the digits is to decompose the number, see:

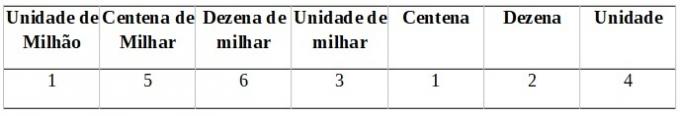
Note that after performing the decomposition, it is possible to determine the relative value of all the digits of the number without much difficulty.

1.563.124

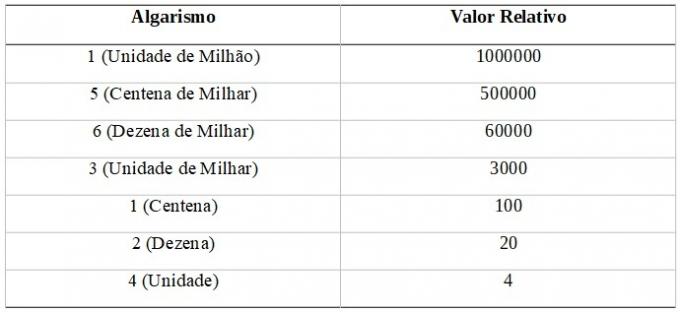
Note that when you add up all the relative values, we get the original number, see:

1.000.000 + 500.000 + 60.000 + 3000 + 100 + 20 + 4 = 1.563.124

See too: Ordinal numbers - numbers that indicate order and position

Example 5 – Determine the absolute and relative values ​​of all the digits of the number 5555.

In order to make it easier, the first step is to decompose the number 5555.

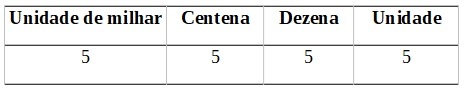
So, notice that the absolute value of all of them is equal to 5. Now the relative value is:

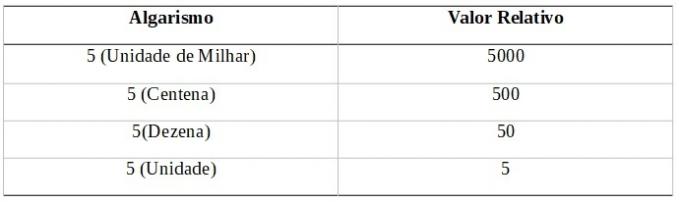
Therefore, we can say that:

5000 + 500 + 50 + 5 = 5555