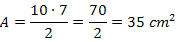One occupation is a rule that relates each element of a set A to a single element of a set B. This rule is usually a algebraic expression, like a equation. Set A is called domain and set B is the counter-domain of the function.
Data a and b belonging to the set of real numbers, with The nonzero, one first degree polynomial function is defined by:
f (x) = ax + b
In this function, x is called the independent variable and f(x) or y is called the dependent variable.
One occupationoffirstdegree, therefore, relates elements of two sets in a way linear. Note, for example, some of the pairs (x, y) obtained in the function y = 2x:
x = 1, y = 2·1 = 2
x = 2, y = 2·2 = 4
x = 3, y = 2·3 = 6
Therefore, they are elements of the domain of that occupation: 1, 2 and 3. And they are elements of the counter-domain of this function: 2, 4 and 6.
Function graph
O graphic is the representation of all the points that belong to a function offirstdegree on the plan. How the function of the first degree is linear, your chart will always be a straight.

First degree function graph
To build it, we must remember one of the postulates of Geometry: there is only one straight which contains two distinct points belonging to the plane.
Using this postulate, it will only be necessary to find the location of two points in the flat to build the straight that contains them. The method used for this depends on the law of formation of the occupationoffirstdegree and will be presented in steps:
1 – Choose two values for x;
2 – Replace these values in the function;
3 – Find the corresponding y values.
Once this is done, the value chosen for x, and its corresponding y, form an ordered pair that can be marked in the Cartesian plane.
Since we choose two values for x, we will have two values for y and therefore two ordered pairs. Knowing that each ordered pair is the location of a Score at the flatCartesian, we already have the two points. So, just mark them and draw the straight that passes through them.
There is a second method to build the graphic that reveals important information about him and that may appear in some exercises. To use it, proceed as follows:
1 – Choose x = 0 and substitute that value into the function to find the related y value. Knowing that the function is y = ax + b, we have the following result:
y = ax + b
y = a·0 + b
y = b
So the first point will be (0, b). This is the meeting point between the graph of the function and the y axis and will always be given by the coefficient b of the occupationoffirstdegree.
2 – Choose y = 0 and substitute this value in occupation to find the related x value. Knowing that the occupationoffirstdegree is y = ax + b, we will have:
y = ax + b
0 = ax + b
ax = - b
x = - B
The
So the second point will be (–b/a, 0). This is sourcegivesoccupation of firstdegree, that is, the meeting point between your graphic and the x-axis.
By doing these two steps, we obtain the coordinates of two points belonging to the graphicgivesoccupation. To build it, just draw the straight that passes through them.
Function Roots
The root, or the zero of a occupationoffirstdegree, is the meeting point between this occupation and the x-axis. To get this point, there are two alternatives:
1 – Design the graphicgivesoccupation and notice where it touches the x-axis.
2 – Make y = 0 and find the value of x related to it.
So, the source gives occupation y = 2x – 8 is:
y = 2x – 8
0 = 2x – 8
2x = 8
x = 8
2
x = 4
Take the opportunity to check out our video classes related to the subject: