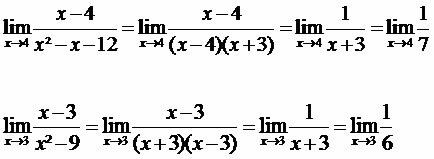Functions, regardless of their degree, are characterized according to the connection between the elements of the sets where the relation is made.
A function A →B can be: surjector, injector, and bijector. To identify these characteristics in a function, it is necessary that we have knowledge of the function definition, of what a domain, image and counter-domain are.
Look at the diagram below that represents a function f: A→B and see who is its domain, image and counterdomain.
Domain will be all elements of set A: D(f) = {-3.1,2,3} the image will be elements of set B that receive the arrow: Im (f) = {1,4,9} and the counterdomain will be all the elements of set B: CD(f) = {1,4,5,9}.
Now, see how to identify these function characteristics:
Overjet function
A function will be surjective if the image set is equal to the counterdomain set, that is, the image set will be all elements of the arrival set. Mathematically, we can say that: f: A →B defined by any formula will be surjective if Im (f) = B.
Injector function
A function will be injectable if the elements of the domain set are linked to distinct images. Mathematically we can say that: f: A → B defined by any formula will be injective if all the elements of A are distinct (different) and the images of those elements are distinct also.
Bijero function
For a function to assume the characteristic of a bijector function, it has to be both surjective and injecting. The image set must be the same as the counterdomain set and all domain elements must be linked to different images.
Do not stop now... There's more after the advertising ;)
by Danielle de Miranda
Graduated in Mathematics
Brazil School Team
Roles - Math - Brazil School
Would you like to reference this text in a school or academic work? Look:
RAMOS, Danielle de Miranda. "Properties of a function"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/propriedades-uma-funcao.htm. Accessed on June 29, 2021.