Pastaspecific is scalar physical greatness which corresponds to the ratio between the mass and volume of a substance. The unit of measurement of the specific mass is kg/m³, however, the use of other units, such as g/cm³ or kg/L, is quite common in the study of hydrostatic, for example. Despite having the same unit of measurement, specific mass and density are different properties.
Lookalso: Mechanical Physics for Enem – what to study?
Specific mass and density
Specific mass and density has the same unit of measurement, both deal with the ratio between mass and volume, however, the specific mass concerns the substance, while the density is related to a body.
A body can be made of iron and still have density less than the Water,floating, as do the big ships. This happens because, despite being massive, the mass of these ships is distributed in a large space, this decreases the density of these bodies.
There is a case where the densityofbody it's the same as pastaspecificgivessubstance that composes it:
when the body is massive. If there are no internal spaces in the body, these two characteristics will be the same.Do not stop now... There's more after the advertising ;)
The formula used to calculate the specific mass of a substance is shown below, check it out:
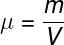
μ – specific mass (kg/m³, g/cm³, kg/L etc.)
m – substance mass
V – volume occupied by the substance
See too: Black holes – discover the densest bodies in the entire Universe
Specific mass and specific weight
Density is different from specific weight. O specific weight of a body concerns the ratio between its weight and volume. The specific weight unit of measure is the N/m³.

Table of specific masses
Below, we present a table with the specific masses of some common substances, note:
Substance |
Specific mass (g/cm³) |
Water |
1,00 |
Ice |
0,92 |
Alcohol |
0,79 |
Iron |
7,85 |
Lead |
11,3 |
Mercury |
13,6 |
Specific mass exercises
Question 1) A solid cube, 3 cm on a side, is made of a material with a specific mass equal to 8 g/cm³. The mass of this cube is equal to:
a) 95 g
b) 216 g
c) 118 g
d) 240 g
Template: Letter B
Resolution:
To calculate the cube's mass, we must find its volume, then use the specific mass formula, since the cube is massive, check it out:
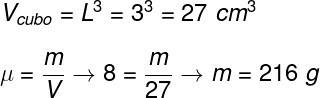
Based on the calculation, the correct alternative is the letter B.
Question 2) Inside a sphere, with a radius of 4 cm, there is a region of hollow volume, with a volume equal to 128 cm³. Knowing that the mass of the sphere is equal to 0.8 kg, the density of the sphere and the specific mass of the substance that composes it are, respectively, equal to:
(Use π = 3)
a) 0.8 g/cm³ and 1.6 g/cm³
b) 3.4 g/cm³ and 1.8 g/cm³
c) 2.5 g/cm³ and 1.25 g/cm³
d) 3.12 g/cm³ and 6.25 g/cm³
Template: Letter C
Resolution:
The first step is to find the total volume of the sphere, after that you can calculate its density easily, just divide the mass of the sphere by the total volume. Then, it is necessary to calculate the massive volume of the sphere, to do this, subtract the total volume by the hollow volume, then just divide the mass by the massive volume, check:

The results obtained are 3.12 g/cm³ and 6.25 g/cm³, therefore, the correct alternative is letter D.