We have that two triangles are congruent:
When its elements (sides and angles) determine the congruence between triangles.
When two triangles determine the congruence between their elements.
Congruence cases:
1st LAL (side, angle, side): two congruent sides and also congruent formed angles.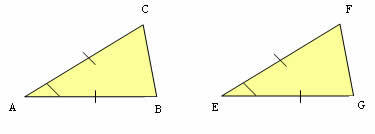
2nd LLL (side, side, side): three congruent sides.

3rd ALA (angle, side, angle): two congruent angles and side between congruent angles.
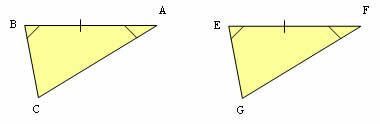
4th LAA (side, angle, angle): congruence of the angle adjacent to the side, and congruence of the angle opposite to the side.
Do not stop now... There's more after the advertising ;)
Through the definitions of congruence of triangles we can arrive at geometric properties without the need to perform measurements. We call this method demo.
We say that in every isosceles triangle the angles opposite the congruent sides are congruent. The base angles of an isosceles triangle are congruent.
by Mark Noah
Graduated in Mathematics
Brazil School Team
See more!
triangles
Properties and elements.
Area of a triangular region
Formulas for calculating the area of a triangle.
plane geometry - Math - Brazil School
Would you like to reference this text in a school or academic work? Look:
SILVA, Marcos Noé Pedro da. "Congruence and similarity of triangles"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/congruencia-e-semelhanca-de-triangulos.htm. Accessed on June 28, 2021.



