Occupation is a rule that relates each element of a set (represented by variable x) to a single element of another set (represented by variable y). For each value of x, we can determine a value of y, then we say that “y it is in function in x”.
Let's represent a function of natural numbers so that for each natural number chosen, we get double it. For example, if we choose the 1, we will have the number 2; if we choose the 2, we will have the 4; if we choose the 3, we will have the 6 and so on. We can represent a function using the arrow diagram or the arrow diagram, as in the following figure:
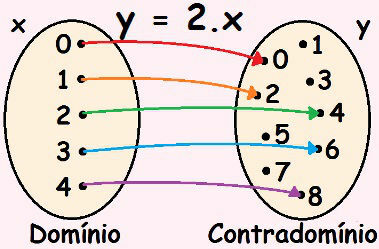
The arrow diagram or arrow diagram is used to represent functions
In this representation there are two numerical sets, a domain and a counterdomain. Inside of counter-domain there is a subset called the Image. This subset is composed of the elements that are receiving the arrow, that is, those that have some relationship with the domain elements. When working with functions, we will always have a “function law
” which will determine how the image elements of that function will look. In this case, there is a function of y in relation to x, since for each x chosen, there is a y. We still say that y and the dependent variable and, in turn, that x and the independent variable.If the domain and image elements of a function belong to the set of integers, for example, we say that f: 
→ , we read that "f is a function whose domain belongs to integers and whose image belongs to integers" or simply, "f is a function of integers in integers".
Do not stop now... There's more after the advertising ;)
Functions can be classified as follows:
-
Overjet function
We say that a function is surjective if all the elements of the counterdomain belong to the set of the image, that is, if all the elements “receive an arrow coming from the domain, or, simply, if the set of image and counter-domain are the same.” The same element of the counterdomain can receive a correspondence from more than one element of the domain.
-
Injector Function
A function is called injector if each element of the domain has a unique and distinct image, that is, an element of the image set can correspond to two elements of the domain.
-
Bijector Function
A function is bijective if it is both surjective and injecting simultaneously, that is, if all the elements of the contradomain belong to the set of the image and an element of the contradomain corresponds to a single element of the domain.
-
Simple Function
A function is said to be simple if it is neither injecting nor surjective.
In the following diagram there is a representation of each type of function using the arrow diagram:
Each type of function has a specific regularity.
By Amanda Gonçalves
Graduated in Mathematics
Would you like to reference this text in a school or academic work? Look:
RIBEIRO, Amanda Gonçalves. "What is function?"; Brazil School. Available in: https://brasilescola.uol.com.br/o-que-e/matematica/o-que-e-funcao.htm. Accessed on June 27, 2021.