Percentage or percentage is an area of mathematics that indicates a calculated rate or proportion against the number 100 (per hundred), and is represented by the % symbol. It consists of a reason that its denominator is always 100.
For example, if in a group of 100 people there are 55 women and 45 men, we can say that the percentage of women is 55%, while the percentage of men is 45%.
Etymologically, the word percentage originated from the Latin percent, which literally means "percent" or "per hundred".
THE percentage is used to compare quantities, calculate profit, discount or loss amounts, and even interest rates.
How to calculate percentage
In mathematics, the calculation of a percentage can be done through the simple rule of 3, as in the examples below.
Example 1
To determine the value of 30% of 200, you must keep in mind that 100% is always equal to the total of units, that is, 200.
The value of units referring to 30% is unknown, this number being "x" the answer obtained with the rule of three.
100% = 200 | 30% = X
So, X over 30 is equal to 200 over 100:
X/30 = 200/100
So we have:
100X = 200.30
We multiply 200 by 30 and get the result of:
100X = 6000
With the result of multiplication, and following the rule of three, we divide the value by 100 to find the value of X.
X = 6000/100
X = 60.
Thus, 30% of 200 is 60.
Example 2
Imagine that a teacher has 450 students and, in the final exams, only 8% of the total of his students got the maximum grade.
To find out how many students got the maximum grade, the percentage calculation must be done as follows:
To find the 8% value of 450, keep in mind that 100% is always equal to the total of units, that is, 450.
The value of units referring to 8% is unknown. So, we'll call it "X" to get the answer through the rule of three:
100% = 450 | 8% = X
X over 8 is equal to 450 over 100:
X/8 = 450/100
Applying the rule of three, we have:
100X = 450.8
So, we multiply 450 by 8 and get the result of:
100X = 3600
With the result of multiplication, and following the rule of three, we divide the value by 100 to find the value of X.
X = 3600/100
X = 36.
So we know that 36 students got the maximum grade in the final exams, because 8% of 450=36.
What is the percentage for?
It is a mathematical measure that is used to compare quantities and determine discounts, additions of values, quantities, etc.
All these calculations are present in everyday life, for example, when you hear phrases:
The price of the basic basket increased 15% compared to last year.
The store will offer discounts of 30% to 70% during the weekend.
The percentage is also often used to determine commissions, that is, an amount of money that is received or paid for some product or service.
It's a word often used in the context of business, because it's the basis of calculation of profits, losses and discounts.
Example:
This year, the company's profits increased 15% compared to the previous year.
Percentage for interest rates
A percentage can also be related to an interest rate.
In the case of simple interest, if a person borrows R$1,000 at a rate of 10% interest per month, and manages to repay the loan after one month, he will have to pay R$1,100.
That is, $1,000 of the money received, plus $100 of the interest (100 is 10% of 1000).
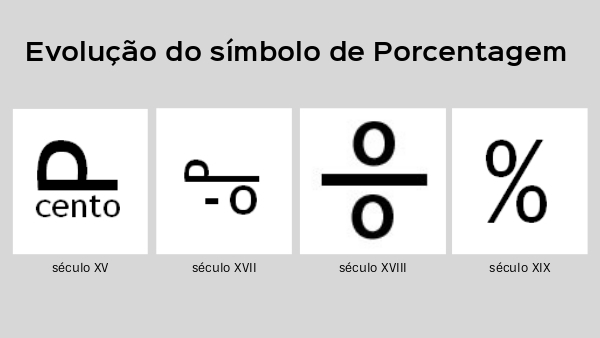
Percent Symbol Origin
The current symbol used to represent the percentage (%) is relatively recent. Some old documents show different ways to indicate the percentage during the Middle Ages, for example.
Initially, the expression "per cent" was used, which quickly evolved into "per 100". Over the centuries, other ways of representing the percentage emerged, such as: pc-o, o/o and finally the %.
What is the percentage point?
The percentage point (pp) consists of the unit that represents the difference between percentages.
For example, when a discount goes from 30% to 45% it means that there has been a 50% increase in the discount amount.
Some people may think the addition is 15%, as this is the percentage that has been added to the initial 30%. However, it is necessary to take into account the fact that 15% represents half of the initial percentage (30%), that is, 50% of this number.
Thus, it can be said that the result between the increase from 30% to 45% is whether 15 percentage points or 50% increase.
