O flattiltedwith frictionis considered a simple machine, as well as being one of the most common and everyday applications of Newton's laws. It is a straight surface, arranged at an oblique angle in relation to the horizontal direction, on which an object is placed that is subject to the action of the forces weight and friction, the latter produced by the compression force, known as normal force, acts between the surface and the body.
For a better understanding of the topic at hand, let's review the ideas of inclined plane and inclined plane friction force. After that, solving exercises that involve inclined planes with friction will enable a good understanding how Newton's three laws should be applied, in particular the fundamental principle gives dynamics.
See too: How to solve exercises on Newton's laws - step by step
inclined plane
inclined plane is a type of simple machine that consists of a surface arranged at an angle to the horizontal direction. In this way, when a body is supported on this surface, the weight force acting on the body in the direction vertical now has a horizontal component, so that the body can slide along the plane, if none other strength act on it.

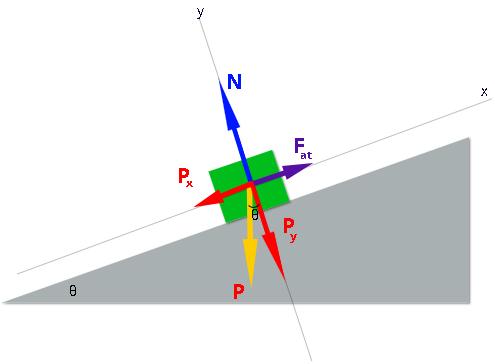
The following figure shows a situation in which a body of mass m is supported on an inclined plane at an angle θ in relation to the x (horizontal) direction. Note that, due to the inclination, the weight force (P) starts to present the P componentsx and Py.

By analyzing the figure, it is possible to see that Px is the opposite side (C.O.) to the angle θ and that Py, consequently, is the adjacent side (C.A) to this angle, for this reason, these components can be written in terms of the functions sine and cosine, in the following way:

Consequently, when solving exercises that involve an inclined plane, it is necessary that the Newton's 2nd Law be applied in both the x and y directions. Therefore, we say that the vector sum of the forces (resulting force), in the x direction and in the y direction, must be equal to the product of the pasta by the x and y components of the acceleration:
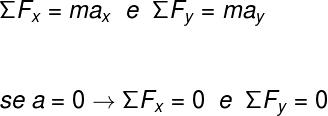
It is important to remember that if the body is at rest or, still, sliding with a constant velocity, then its acceleration will necessarily be equal to 0, according to the Newton's 1st law, the law of inertia.
Friction force on the inclined plane
The friction force (Funtil) arises when there is contact between surfaces that are not perfectly smooth, this force has originmicroscopic and is proportionalto the compression force that one body exerts on the other, known as normal strength.
The formula used to calculate the friction force is shown below, check it out:
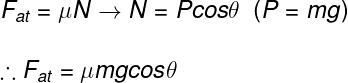
μ - coefficient of friction
m – mass (kg)
g – gravity (m/s²)
In the previous image, it is also shown that the strengthnormal No, at least in most of the exercises, equal to the y component of the weight, this is true whenever there are no forces other than the weight and normal forces acting in the y direction.
There are two cases of friction force, the static friction force and the dynamic friction force. The first case applies to the situation in which the body is at rest, the second is related to the situation in which the body slides on the inclined plane.
The force of static friction is always proportional to the force that tries to put the body in motion, and by this, this one increases in the same proportion as that, until the body starts to slide on the plane tilted. In this case, to calculate the friction force, we must use the coefficientinfrictiondynamic, which always has a lower value than the static friction coefficient.
Remember that the friction force always acts in the opposite direction from which the body slides on the inclined plane, and this affects the algebraic sign assigned to it during solving according to the positive orientation of the x and y directions.
See too: Free fall - what is it, examples, formula and exercises
Inclined plane with friction
The friction inclined plane, in its simplest form, involves the action of weight force and friction force. There is threesituations which can be considered in this regard: a first, in which the body is static; The Monday, when the body slides with constant speed; and the third, in which the body slides in an accelerated way.
At the first and second case, the net force in the x and y directions is nil. What sets them apart, in fact, is just the coefficient of friction, which, in the first case, is static, and, in the second, is dynamic. In the last case, the coefficient of dynamic friction is used, however, the resulting force is non-zero and, therefore, is equal to the mass of the body multiplied by the acceleration.
In order to put into practice and better understand the theory of the inclined plane with friction, it is necessary to solve some exercises, shall we?
See too: The most important topics of mechanical physics for Enem
Exercises solved on an inclined plane with friction
Question 1) (UERJ) A block of wood is balanced on a 45º inclined plane in relation to the ground. The intensity of the force that the block exerts perpendicularly to the inclined plane is equal to 2.0 N. Between the block and the inclined plane, the intensity of the friction force, in newtons, is equal to:
a) 0.7
b) 1.0
c) 1.4
d) 2.0
Template: letter D
Resolution:
The statement states that the block is in equilibrium, this means that the resulting force on it should be equal to 0, moreover, the normal force between the block and the inclined plane is equal to 2.0 N. Based on this information, the exercise asks us to calculate the intensity of the friction force.
If, in this resolution, we used the friction force formula indiscriminately, we would realize that some data were not informed by the statement, such as the coefficient of static friction, moreover, we would be making a mistake, since this formula would allow we calculate the maximum value of the static friction force and not the static friction force that is necessarily being exerted on the block.
Therefore, to solve the exercise, it is necessary to realize that, once the block is stopped, the forces in the x direction, the one that is parallel to the inclined plane, cancel out, therefore, the weight component in the x direction (Px) and the friction force, which is opposite to this component, have equal modules, check:

After having considered the vector sum of the x and y directions, we started to solve the expressions obtained in the red color, observe:

In the previous calculation, we found out what the weight P of the body was, then based on the equality between the force. of friction and Px, we calculate the value of this force, which is equal to 2.0 N, so the correct alternative is the letter D.
Question 2) (PUC-RJ) A block slides from rest down an inclined plane that makes an angle of 45° with the horizontal. Knowing that during the fall, the acceleration of the block is 5.0 m/s² and considering g = 10 m/s², we can say that the coefficient of kinetic friction between the block and the plane is:
a) 0.1
b) 0.2
c) 0.3
d) 0.4
e) 0.5
Template:
Resolution:
To solve the exercise, we must apply Newton's 2nd law in the x and y directions. Let's start by doing this for the x direction, so we must remember that the net force in this direction must be equal to the mass times the acceleration:

After replacing Px and Funtil, we simplify the masses present in all terms, then we reorganize these terms, so that the friction coefficient was isolated, then we substituted the values in the obtained formula and applied The distributive property in the last step, obtaining a value equal to 0.3 for the friction coefficient, therefore, the correct alternative is the letter c.
By Rafael Hellerbrock
Physics teacher
Source: Brazil School - https://brasilescola.uol.com.br/fisica/plano-inclinado-com-atrito.htm
