O Pythagorean theorem is expression math that relates the sides of a right triangle, known as hypotenuse and peccaries. That theorem it is not valid for sharp or obtuse triangles, just for rectangles.
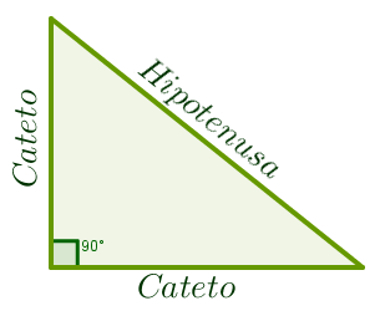
for a triangle be considered rectangle, just that one of your angles have a measure equal to 90°, that is, that the triangle has a right angle. The side opposite this angle is the longest side of the right triangle and is called the hypotenuse. The other two smaller sides are called the peccaries, as shown in the following figure:
Mathematical expression: Pythagorean Theorem
The square of the hypotenuse is equal to the sum of the squares of the legs.
That expression it can also be represented in the form of an equation. For this, do hypotenuse = a, collar 1 = b and collared 2 = c. Under these conditions, we will have:
The2 = b2 + c2
This is a valid formula for the following triangle:

Mind Map: Pythagoras Theorem
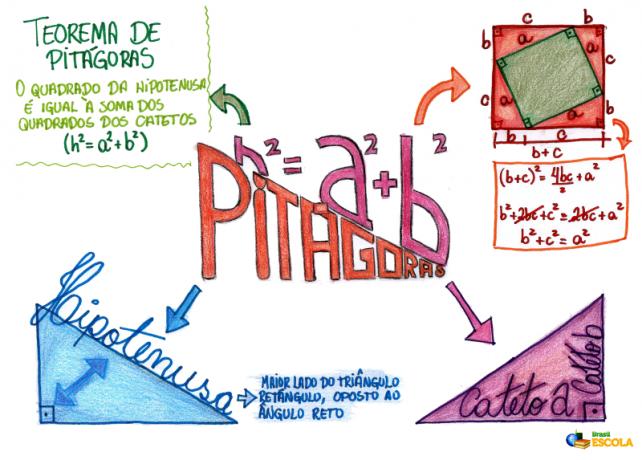
*To download the mind map in PDF, Click here!
Example
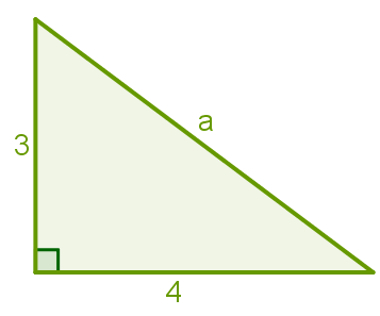
1.Calculate the measurement of hypotenuse of trianglerectangle present in the following figure.
Solution:
Note that 3 cm and 5 cm are the measurements of the peccaries of triangle above. The other measurement refers to the side opposite the right angle, so the hypotenuse. Using the theorem in Pythagoras, we will have:
The2 = b2 + c2
The2 = 42 + 32
The2 = 16 + 9
The2 = 25
a = √25
a = 5
The hypotenuse of this triangle measures 5 centimeters.
2. The side opposite the right angle of a right triangle measures 6 inches and one of the other two sides measures 12 inches. Calculate the measurement of the third side.
Solution:
The side opposite the right angle is the hypotenuse. The other two are cocky. Representing the missing leg by the letter b, we can use the theorem in Pythagoras to discover the third measure. Just remember that she is also a collared one. Therefore, we will have:
The2 = b2 + c2
152 = b2 + 122
Note that the measurement of hypotenuse was placed in place of the letter a, as this letter represents that measurement. Solving the equation, we will find the value of b:
225 = b2 + 144
225 - 144 = b2
81 = b2
B2 = 81
b = √81
b = 9
The third side measures 9 centimeters.
3. (Enem 2006) In the figure below, which represents the design of a staircase with 5 steps of the same height, the total length of the handrail is equal to:

a) 1.8 m.
b) 1.9 m.
c) 2.0 m.
d) 2.1 m.
e) 2.2 m.
Solution:
Note the following trianglerectangle on the handrail of the exercise image.

Note that the length of the handrail is equal to the sum of 30 + a + 30 and that "a" is the measure of the hypotenuse of the triangle placed over the image. Also note that b = 90 and that c = 24 + 24 + 24 + 24 + 24 = 120. So, to find out the measure of a, we will do:
The2 = b2 + c2
The2 = 902 + 1202
The2 = 8100 + 14400
The2 = 22500
a = √22500
a = 150 centimeters.
The measurement of the handrail is 30 + 150 + 30 = 210 cm or 2.1 m.
Template: letter D.
By Luiz Paulo Moreira
Graduated in Mathematics
Source: Brazil School - https://brasilescola.uol.com.br/o-que-e/matematica/o-que-e-teorema-de-pitagoras.htm


