THE potentiation it is a simplification of how to expose a multiplication of equal factors. Before detailing enhancement, let's remember addition. In the early grades, we learn to add and soon we see that there are ways to better express sums, such as:
a) 2+2+2+2+2+2+2
b) 3+3+3+3+3
c) 4+4+4+4+4+4+4+4+4+4
In the item The, if we add the number 2 to itself 7 times, we get the result 14. But this result could have been obtained more quickly by calculating 2 x 7 = 14. In the item B, the sum of the number 3 five times can be replaced by the multiplication of 3 x 5, because in both we obtain the result 15. In the item ç, the sum of the number 4 ten times can be represented by the multiplication of 4 x 10, which is equal to 40.
Just as we can express a sum of equal factors through the product of that factor by the number of times it is repeated, we can substitute the multiplication of terms for the potentiation. Let's look at an example:
3 x 3 = 9
3 x 3 x 3 = 27
3 x 3 x 3 x 3 = 81
In the three examples above, we are just multiplying the number 3
. Now let's see what multiplication would look like by repeating the number 3 ten times.3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 = 59,049
To simplify the notation of these multiplications, we can use potentiation. This form of representation was originally created by the mathematician and philosopher René Descartes (1596 – 1650). In potentiation, we represent only once the number that will be multiplied and, above that number, we put the number of times it will be repeated. For the examples above, let's see how the representation through enhancement will look:
3 x 3 = 32
3 x 3 x 3 = 33
3 x 3 x 3 x 3 = 34
3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 = 310
Do not stop now... There's more after the advertising ;)
We can generalize the representation of a power as follows, whether The and B rational numbers, then:
The x The x The x... x The = TheB
Btimes
As with other operations, the terms of a power are given specific names:
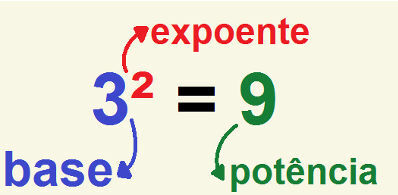
The terms of a potentiation are the base, the exponent and the potency
The reading of a power also takes place in a particular way. The example above reads as "three to two", "three to the second power" or, more popularly, "three squared" or "three squared". When it comes to exponent three, there is also a specific variation. The potency can be read as "cubed". Only exponents two and three have these variations, the reading of the rest of the exponents follows the same idea. See the examples below:
24 = "two to the four" or "two to the fourth power"
25 = "two to the five" or "two to the fifth power"
26 = "two to the six" or "two to the sixth power"
27 = "two to the seven" or "two to the seventh power"
28 = "two to the eight" or "two to the eighth power"
29 = "two to the nine" or "two to the ninth power"
2no = "two to the no” or “two to the umpteenth potency"
In general, when we are faced with a power, we need to repeat the product of the base as many times as the exponent. But three rules are easily seen:
-
When the base is zero, the power result will be zero.
0no = 0
-
When the exponent is a, the power result will be exactly the base value.
The1 = the
-
When the exponent is zero, the power result will always be a.
The0 = 1
By Amanda Gonçalves
Graduated in Mathematics
Would you like to reference this text in a school or academic work? Look:
RIBEIRO, Amanda Gonçalves. "What is enhancement?"; Brazil School. Available in: https://brasilescola.uol.com.br/o-que-e/matematica/o-que-e-potenciacao.htm. Accessed on June 27, 2021.
