Speedvector it is the measure with which a certain distance is covered, during a period of time, when we take into account vector parameters, such as magnitude, direction and direction. The velocity vector can be calculated by the displacement vector — difference between the vectors of final and initial position — divided by the time interval in which the movement took place.
Lookmore: Static equilibrium: when the resultant of forces and the sum of torques are null
Definition of vector velocity
unlike speed climb, the average vector velocity it may be null, even if the body is in motion. This happens in cases where the mobile starts from a position and, at the end of a certain period of time, returns to the same position. In this case we say that, even if the space traversed by the rover was not null, the vector displacement was.movement.can be null, even if the body is in, the average vector velocity climbunlike speed
The formula used to calculate the velocityvector from some furniture is this:

v – vector velocity
S - vector displacement
t - time interval
vector displacement
we call sF and s0, respectively, the positions in which the mobile was at the end and beginning of the movement. These positions can be written in the form of points of Cartesian plane(x, y), so we can calculate vector displacement, taking into account the distance between the x and y coordinates of each of the points.
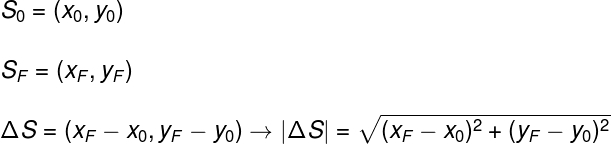
Another way to write the displacement vector is through the use of vectorsunitary (a vector that points in the x, y, or z directions and has a modulus of 1). Unit vectors are used to define the magnitude of each component of displacement or velocity in directionshorizontal and vertical, represented by the symbols i and j, respectively.
In the following figure, we will show the components of the displacement vector of a mobile that was in the position s0 = 4.0i + 3.0j, and then moves to position sF = 6.0i and 10.0j. The displacement, in this case, is given by the difference between these positions and is equal to ΔS = 2.0i + 7.0j.

knowing the velocity vector components, it is possible to calculate the moduleofdisplacement, for that, we must use the Pythagorean theorem, since these components are perpendicular to each other, note:

After we find the magnitude of the displacement vector, the vector speed can be calculated by dividing it by the time span.
see more: Force: agent of dynamics responsible for changing the state of rest or movement of a body
vector velocity and scalar velocity
As mentioned, velocity is a vector quantity, so it is defined based on its magnitude, direction, and direction. All speed is vector, however, most textbooks use the term “scalar speed” to facilitate the study of kinematics for high school students. That said, this “climb” speed it is actually the magnitude of the velocity of a rover moving along a single direction in space.
Average and instantaneous velocity
Average velocity is the ratio between the vector displacement and the time interval in which this displacement occurs. When we calculate the average speed, the result obtained does not indicate that it was maintained throughout the journey, and may have suffered variations over time.
THE instantaneous velocity, in turn, is set to breaksintimeinfinitesimal, ie very small. The definition of instantaneous velocity refers, therefore, to the measuregivesvelocityineachinstant:
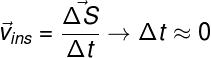
Exercises on vector speed
Question 1) (Mackenzie) A plane, after traveling 120 km to the northeast (NE), moves 160 km to the southeast (SE). With a quarter of an hour being the total time of this trip, the modulus of the average vector speed of the plane, in that time, was:
a) 320 km/h
b) 480 km/h
c) 540 km/h
d) 640 km/h
e) 800 km/h
Template: Letter e
Resolution:
The north and northeast directions are perpendicular to each other, so we will calculate the vector displacement of this plane using Pythagoras' theorem. Note the following figure that illustrates the situation described and the calculation to be performed initially:

After calculating the modulus of the vector displacement, just calculate the average vector velocity, dividing it by the time interval, which is ¼ of an hour (0.25 h):

Based on this, we find that the plane's speed is 800 km/h, so the correct alternative is the letter e.
Question 2) (Ufal) The location of a lake, in relation to a prehistoric cave, required walking 200 m in a certain direction and then 480 m in a direction perpendicular to the first. The distance in a straight line, from the cave to the lake, was, in meters,
a) 680
b) 600
c) 540
d) 520
e) 500
Template: Letter D
Resolution:
The exercise talks about two perpendicular displacements. To find the distance between the final and initial points, we must use the Pythagorean theorem, note:

According to the result obtained, the correct alternative is the letter d.
Question 3) (Uemg 2015) Time is a flowing river. Time is not a clock. He is so much more than that. Time passes whether you have a watch or not. A person wants to cross a river in a place where the distance between the banks is 50 m. To do this, she orients her boat perpendicular to the shore. Assume that the boat speed in relation to the water is 2.0 m/s and that the current has a speed of 4.0 m/s. About crossing this boat, tick the CORRECT statement:
a) If the current did not exist, the boat would take 25 s to cross the river. With the current, the boat would take more than 25 s to cross.
b) As the boat speed is perpendicular to the banks, the current does not affect the crossing time.
c) The crossing time, under no circumstances, would be affected by the current.
d) With the current, the boat crossing time would be less than 25 s, because it vectorial increases the boat speed.
Template: Letter C
Resolution:
Regardless of the current speed, the boat's crossing time will be the same, as it crosses perpendicularly to the banks.

Understand: the composition of the two speeds of the boat causes it to move in the direction resulting from them, thus the direction perpendicular to the river, which is 50 m long, is always covered by the speed of the boat, which is 2.0 m/s, and, therefore, the crossing time is not affected.
By Rafael Hellerbrock
Physics teacher
Source: Brazil School - https://brasilescola.uol.com.br/fisica/velocidade-vetorial.htm


