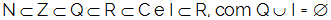You numerical sets they are meetings of numbers that have one or more characteristics in common. all setnumeric It has subsets, which are defined by imposing an additional condition on the observed numerical set. This is how the sets of numberspairs and odd, which are subsets of the whole numbers.
For this reason, it is important to understand well what they are sets, subsets and the set of numberswhole for more in-depth details on the numbers pairs and odd.
whole numbers set
O set From numberswhole it is formed only by numbers that are not decimals, that is, they do not have a comma. In other words, they are numbers that represent units that have not yet been split.
To this set belong the numberswhole negative, zero and positive integers. So, we can write its elements as follows:
Z = {…, – 3, – 2, – 1, 0, 1, 2, 3, …}
An additional information: the set of numbersnatural is contained in the set of whole numbers, since natural numbers are those that, in addition to integers, are not negative. Therefore, the set of natural numbers is one of subsets of the set of numberswhole.
Pair numbers
As well as the set From numbersnatural is a subset of numberswhole, the set of numbers pairs it's also. At first, we learn to recognize the elements of the set of even numbers through play. The rule used is: all even number ends with 0, 2, 4, 6 or 8. So 224, for example, is an even number because it ends with the digit 4.
However, this is a consequence of the formal definition of numberpair, which can be understood as:
Every even number is a multiple of 2.
There are other definitions for the elements of this subset From numberswhole, for example:
Every even number is divisible by 2.
The "algebraic definition" used to recognize the elements of this set is: given a number p, belonging to the set of numberswhole, p will be pair if:
p = 2n
In this case, n is an element of the set of numberswhole. Note that this is the "translation" of the first definition in algebraic terms.
Odd numbers
You numbersodd are the elements of the set of numberswhole that are not pairs, that is, numbers that end with any of the digits 1, 3, 5, 7 or 9. Formally, the set of odd numbers is a subset of the integers, and the definition of its elements is:
Every odd number is not a multiple of 2.
The elements of this subset can still be defined:
Do not stop now... There's more after the advertising ;)
Every odd number is not divisible by 2.
In addition, it is also possible to write the algebraic definition for the elements of the set of numbersodd: given an integer i, it will be odd if:
i = 2n + 1
In this definition, n is a number belonging to the set of numberswhole.
properties
The following properties are a result of defining numberspairs and odd and the ordering of the set of numberswhole.
1 - Between two numbersodd consecutives there is always one numberpair.
That's why there need be no doubt about the number zero. As it is between – 1 and 1, which are integers odd consecutive, so he is pair.
2 – Between two numbers pairs consecutive there is always a number odd.
3 – The sum between two consecutive whole numbers will always be one numberodd.
To show this, consider n a numberwhole and note the addition between 2n and 2n + 1, which are the consecutive integers formed by it:
2n + 2n + 1 =
4n + 1 =
2(2n) + 1
Knowing that 2n is equal to the integer k, we have:
2(2n) + 1 =
2k + 1
Which falls precisely under the definition of numberodd.
4 – Given consecutive numbers a and b, a is even and b is odd, the difference between them will always be equal to:
1, if a < b
– 1, if a > b
As the numbers are consecutive, the difference between them must always be one unit.
5 – The sum between two numbersodd, or between two numbers pairs, results in a number pair.
Given the numbers 2n and 2m + 1, we will have:
2n + 2n = 4n = 2(2n)
Making 2n = k, which is also a numberwhole, we will have:
2(2n) = 2k
which is a numberpair.
2m + 1 + 2m + 1 = 4m + 2 = 2(2m + 1)
Knowing that 2m + 1 = j, which is also a numberwhole, we will have:
2(2m + 1) = 2j
which is a numberpair. Using similar calculations, we can complete all of the following properties:
6 – The sum between a numberpair it is a numberodd is always equal to an odd number.
7 – The difference between two numbersodd, or between two numbers pairs, is always equal to an even number.
8 – The product between two numbersodd is equal to an odd number.
9 – The product between two even numbers will result in a number pair.
By Luiz Paulo Moreira
Graduated in Mathematics