The equations that can be solved in the form sin x = sin a. This equation means that if we find two angles that have the same sine, then their sum must be 180°.
Where x is the unknown of the equation and The is the other angle that can be represented in radians that has the same sine as x.
The solution to this equation is done as follows:
S = {x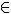 
R ׀ x = a + 2kπ or x = π – a + 2kπ}
See below the resolution of a trigonometric equation using the fundamental trigonometric equation sin x = sin a.
Example:
To find the solution set of the equation sin x = 1 it is necessary to have the knowledge of
2
some concepts in trigonometry.
First we must find what angle can be put in place of x so that the cosine is equal to 
.
Observing the table of notable angles trigonometric functions we see that sin of 30° is equal to .
We pass 30° to radians, using the rule of three: 180° is
for π just like 30° is for π.
6
by Danielle de Miranda
Graduated in Mathematics
Brazil School Team
Trigonometry - Math - Brazil School
Source: Brazil School - https://brasilescola.uol.com.br/matematica/resolucao-1-equacao-fundamental-1.htm
