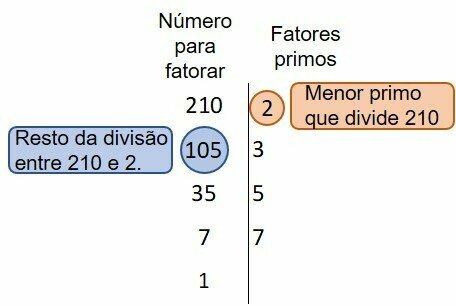
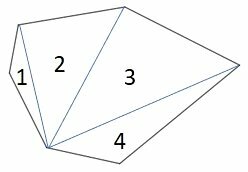
Notable products are multiplications between binomials very frequent in Mathematics, involving algebraic calculations. The products between the best known binomials are:
sum square between two terms
(a + b) ² = a² + 2ab + b²
Square of the difference between two terms.
(a – b) ² = a² – 2ab + b²
Cube of the sum between two terms.
(a + b) ³ = a³ + 3a²b + 3ab² + b³
Cube of the difference between two terms.
(a - b) ³ = a³ - 3a²b + 3ab² - b³
Product of the sum for the difference.
(a + b) * (a - b) = a² - b²
Special cases are as follows:
Sum square of three terms
(a + b + c) ² = (a + b + c) * (a + b + c) = a² + ab + ac + ab + b² + bc + ac + bc + c² = a² + b² + c² + 2ab + 2ac + 2bc
In this case, we are able to apply the following practical rule:
Do not stop now... There's more after the advertising ;)
The sum of,
The square of the 1st term.
The square of the 2nd term.
The square of the 3rd term.
Double the 1st term for the 2nd term.
Double the 1st term for the 3rd term
Double the 2nd term for the 3rd term.
The following multiplications are also considered special cases, as the resolution can be performed by applying a rule of thumb.
(a + b) * (a² - ab + b²) = a³ - a²b + ab² + a²b - ab² + b³ = a³ + b³
(a - b) * (a² + ab + b²) = a³ + a²b + ab² - a²b - ab² - b³ = a³ - b³
Creating new rules of thumb related to the development of certain notable products is an open branch in Mathematics. In this way, by manipulating algebraic terms, we can create new practical rules for solving algebraic situations.
by Mark Noah
Graduated in Mathematics
Brazil School Team
Notable products - Math - Brazil School
Would you like to reference this text in a school or academic work? Look:
SILVA, Marcos Noé Pedro da. "Special Cases Involving Notable Products"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/casos-especiais-envolvendo-produtos-notaveis.htm. Accessed on June 29, 2021.