A fact much discussed is the use of the concepts of matrices and determinants in entrance exams. In this regard, it is necessary to study and understand in what ways these concepts are usually charged in the various entrance exams.
The part of matrices is quite extensive, as it has a differentiated and particular arithmetic system, among other new concepts that are used only in the numerical group of matrices. Therefore, it is important to understand the arithmetic concepts (addition, subtraction, multiplication), consequences arising from the arithmetic system (transposed matrix, inverse matrix) and the determinants of matrices, concepts that can be studied in section Matrix and Determinant.
Something that is observed in the entrance exams is that the matrices are a minority in the questions and when they appear in the entrance exam, almost all concepts about matrices are demanded in a single question. In this article, we'll show you how these questions are addressed, and we'll see how to relate array concepts into a single question.
We must pay attention to the conception of the issues that are addressed regarding their interdisciplinarity, which corroborates their application in a real context. Therefore, we will face issues that need an interpretation and understanding of the statement so that we can determine what should be answered and what information the statement offers.
Question 1) (Faap-SP) An automaker produces three vehicle models, A, B and C. Two types of air bags, D and E. The matrix [air bag model] shows the number of units of air bags installed:

In a given week, the following quantities of vehicles were produced, given by the matrix [model-quantity]:
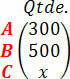
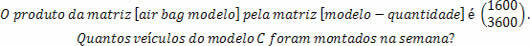
a) 300 c) 150 e) 100
b) 200 d) 0
Resolution: The question involves three matrices, a matrix that lists the number of air bags in each of the three models produced by the factory, the matrix that informs the number of cars produced per week, and the matrix product of these two matrices cited.
The ultimate goal is to determine the number of Model C cars assembled during the week. This quantity is expressed by the unknown x. To determine the unknown value x, we must assemble this matrix equation.
For practicality in notation, we will denote matrices as follows:
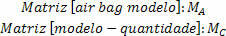
Therefore, we have the following expression:
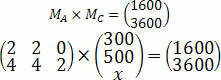
Do not stop now... There's more after the advertising ;)
At this point, we must understand the concepts of matrix equations – these concepts need to understand the arithmetic operations of matrices and matrix equality.

Note that the first line corresponds to the number of cars produced with the air bag type D; and the second line, the number of cars produced with air bag of type E. However, note that no model C car was manufactured using the air bag D. With that, we just need to determine the number of model C cars with the air bag And, that is, we will use the second line.

2) (UEL - PR) One of the ways to send a secret message is through mathematical codes, following the steps:
1. Both recipient and sender have a C key array;
2. The recipient receives a matrix P from the sender, such that MC=P, where M is the message matrix to be decoded;
3. Each number in matrix M corresponds to a letter of the alphabet: 1=a, 2=b, 3=c,..., 23=z;
4. Let's consider the 23-letter alphabet, excluding the letters, k, w, and y.
5. The number zero corresponds to the exclamation point.
6. The message is read, finding the matrix M, matching number/letter and sorting the letters by rows of the matrix as follows: m11m12m13m21m22m23m31m32m33.
Consider the matrices:

Based on the knowledge and information described, mark the alternative that presents the message that was sent through matrix M.
a) Good luck! b) Good proof! c) Boatarde!
d) Help me! e) Help!
Resolution: We must pay attention to the matrix equation that encodes/decodes the message. MC=P, it will be the basis for our calculations.
The matrices C and P were informed, the matrix M is what we want to discover, so we will determine its elements as unknowns equal to what was informed in the sixth step given in the statement.

Equating the elements of the two matrices we will be able to obtain the values of the elements of the matrix M.
m11=2; m12= 14; m13=1; m21=18; m22=14; m23=17; m31=19; m32=5; m33=0.
Transposing to letters we obtain: Good luck!
Note that, as many concepts are covered, attention is needed in the operations between matrices, as there are several operations at the same time. With care and organization, issues involving matrices will not be a hindrance in your entrance exam.
By Gabriel Alessandro de Oliveira
Graduated in Mathematics
Brazil School Team
Would you like to reference this text in a school or academic work? Look:
OLIVEIRA, Gabriel Alessandro de. "Application of matrices in the vestibular"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/aplicacao-das-matrizes-nos-vestibulares.htm. Accessed on June 29, 2021.