A multiplication table is a table that organizes the basic operations: addition, subtraction, multiplication and division. To learn these operations and their results, it is not necessary to memorize the multiplication table, but rather to discover how it works. This means knowing some relationships and properties of mathematical operations.
Read too: What does the remainder of the division mean?
Summary about the multiplication table
- The basic mathematical operations are addition, subtraction, multiplication and division.
- The organization of these operations in tables are the multiplication tables.
- The multiplication tables can be used as a support for learning operations.
- The Cartesian multiplication table is another organization of the multiplication table.
- Addition and subtraction are inverse operations, and multiplication and division are also inverse operations.
- The commutative property is valid for addition and multiplication operations.
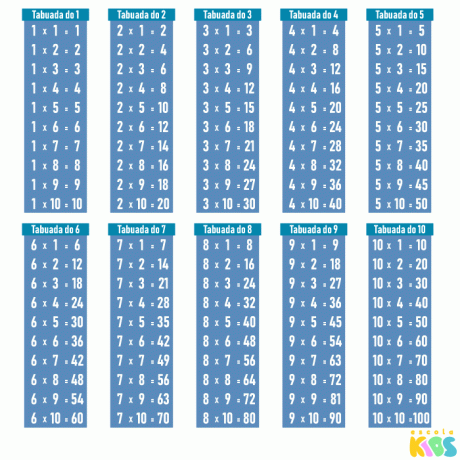
Addition times table
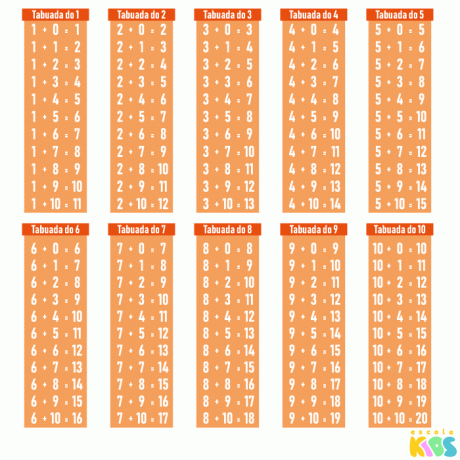
Subtraction table
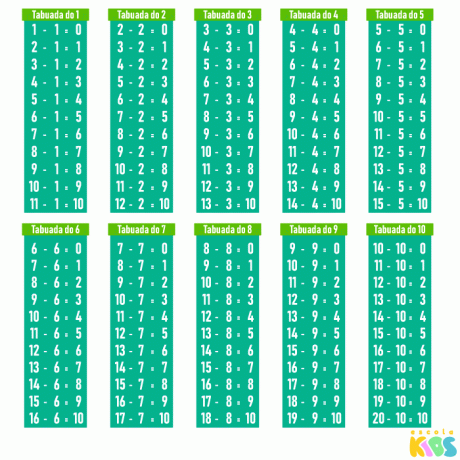
Multiplication table
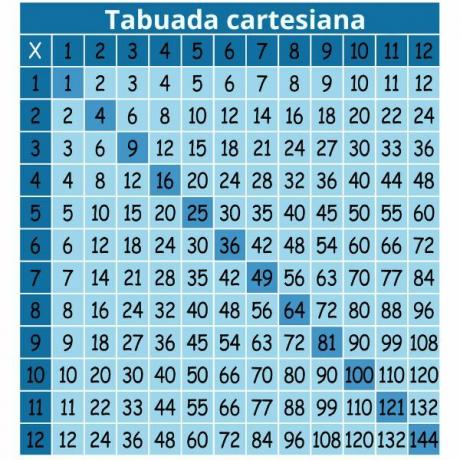
Cartesian multiplication table
The multiplication table is an arrangement of the multiplication tables of multiplication. In the first line and first column of this table, we write the factors (starting from 1) that we want to multiply. In the example below, the factors are 1 to 12. From that, At the intersections of this multiplication table, we write the result of the multiplication between the respective row and column numbers.
Division table
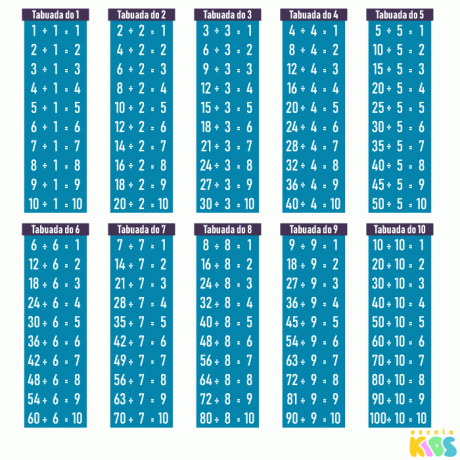
See too: Infallible tip for learning the 9 multiplication table
Tips for learning the multiplication tables
The main tips for learning the multiplication tables are: know the relationships between basic mathematical operations and know their properties. First, let's learn about the relationships between operations.
- Tip 1: The subtraction operation is the inverse of the addition operation.
Consider the operations below:
3 + 4 = 7
7 - 4 = 3
Note that in the first operation we started with the number 3, added 4 and got the number 7 as the answer. In the second operation we started with the number 7 (result of the first operation), subtracted 4 and got 3 as the answer (which was the number we started with).
Do you realize that there is a relationship between the first and second operation?
The second operation (subtraction) undid what the first (addition) had done. Therefore, addition and multiplication are inverse operations.
Let's look at other examples:
a) 9 + 1 = 10 and 10 – 1 = 9
b) 2 + 6 = 8 and 8 – 6 = 2
c) 5 – 2 = 3 and 3 + 2 = 5
- Tip 2: The division operation is the inverse of the multiplication operation.
Consider the operations below:
2 × 3 = 6
6 ÷ 3 = 2
Applying the same reasoning as the previous tip, we conclude that multiplication and division are inverse operations.
Let's look at other examples:
a) 7 × 5 = 35 and 35 ÷ 5 = 7
b) 10 ÷ 2 = 5 and 5 × 2 = 10
c) 4 × 10 = 40 and 40 ÷ 10 = 4
Now let's get to know some properties of operations.
- Tip 3 (commutative property of addition): In addition operation the order of the installments does not change the sum, and in the multiplication operation, the order of the factors does not change the product.
Analyze the numbers and operations below, referring to the addition tables:
6 + 4 = 1 0 and 4 + 6 = 10
1 + 4 = 5 and 4 + 1 = 5
Note that changing the order of the added numbers did not change the result. This property is called commutative property of addition.
Careful! This property is not valid for the subtraction operation:
7 - 1 = 6, but 1 - 7 = -6
- Tip 4 (commutative property of multiplication): In the addition operation, the order of the installments does not change the sum, and in the multiplication operation the order of factors does not change the product.
Examine the numbers and operations below, referring to the multiplication tables:
3 × 4 = 12 and 4 × 3 = 12
7 × 2 = 14 and 2 × 7 = 14
Note that changing the order of the multiplied numbers did not change the result. This property is called commutative property of multiplication.
Careful! This property is not valid for the division operation:
15 ÷ 3 = 5, but 3 ÷ 15 = 0.2
- Tip 5 (neutral element property of addition and subtraction): Addition or subtraction between a number and 0 results in the number itself.
3 + 0 = 3
9 - 0 =
O 0 is called neutral element addition and subtraction operations, as it does not influence the result.
- Tip 6(property of the neutral element of multiplication):
10 × 1 = 10
8 ÷ 1 = 8
1 is called the neutral element of multiplication and division operations, as it does not influence the result.
multiplication table games
Test your knowledge in a game of addition and subtraction tables. Fill in the blanks with the addition operation symbol + or the subtraction operation symbol –.
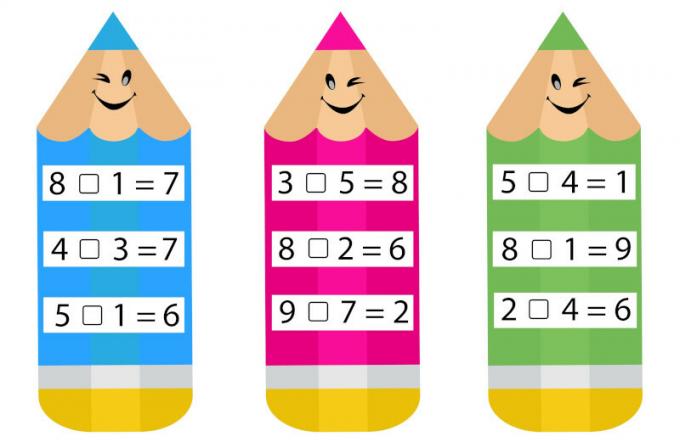
Check out your answers below!
In blue pencil:
8 - 1 = 7
4 + 3 = 7
5 + 1 = 6
In pink pencil:
3 + 5 = 8
8 - 2 = 6
9 - 7 = 2
In green pencil:
5 - 4 = 1
8 + 1 = 9
2 + 4 = 6
Know more: How to divide with a comma
Solved exercises on multiplication tables
Question 1
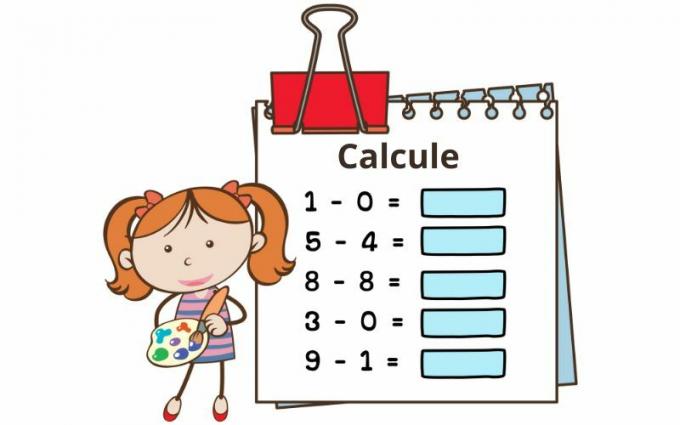
Which numbers fill in the gaps from top to bottom?
a) 1, 1, 0, 3 and 8.
b) 1, 1, 8, 0 and 9.
c) 0, 4, 0, 3 and 1.
d) 0, 5, 0, 3 and 9.
e) 0, 1, 8, 3 and 9
Resolution
1 - 0 = 1
5 - 4 = 1
8 - 8 = 0
3 - 0 = 3
9 - 1 = 8
Alternative A.
Question 2

Using the 2 multiplication table, indicate which numbers fill the gaps from top to bottom.
a) 2, 7, 10, 2 and 1.
b) 4, 2, 10, 2 and 3.
c) 2, 1, 1, 4 and 3.
d) 1, 2, 10, 4 and 2.
e) 2, 2, 2, 2 and 2.
Resolution
Analyzing the multiplication table for 2, it follows that the numbers that fill the gaps from top to bottom are 4, 2, 10, 2 and 3.
Alternative B.
Sources
COSTA, G. O. from the. The multiplication table in the process of teaching and learning mathematics. Course Completion Work (Degree in Mathematics) – State University of Amazonas. Parintins, 2020. Available in: http://repositorioinstitucional.uea.edu.br/handle/riuea/3404.
HOLANDA, K. H. W. in. New perspective for teaching multiplication tables: traces of a diagnostic investigation between teachers and students. Course Completion Work (Degree in Mathematics) – Federal University of Alagoas. Arapiraca, 2017. Available in: https://ud10.arapiraca.ufal.br/repositorio/publicacoes/965.



