Before we get into these concepts, let's discuss what characterizes an equation. In it we come across three important elements (operations, equality and unknown), so that the we relate these three elements, we will seek to determine the value of the unknown that satisfies that equality. This conception continues for Matrix Equations, with only one caveat: unknowns are matrices.
In order for this study to be fully understood, it is advisable that you review the topics on Addition and subtraction of matrices , Matrix multiplication and Multiplying a real number by an array.
We will see some resolutions of matrix equations so that we can understand the process carried out to obtain the solution matrix.
Example 1
Find the matrix X, which satisfies the following equality X-A=B, Where
Before we start using matrices, we will use the given equality to isolate our unknown X.
Therefore, we will substitute the matrices we know in this equation in order to find the matrix X.
Do not stop now... There's more after the advertising ;)
Example 2
If it is possible to solve matrix equations, why not systems of matrix equations? Let's look at an example:
Determine the matrices X and Y, which satisfies the following system.
First, we must find the relations of X and Y, through the given system, and then start the calculation of each matrix.

Therefore, we have two relations for the solution matrices.
Finding the Y matrix:

Finding matrix X:
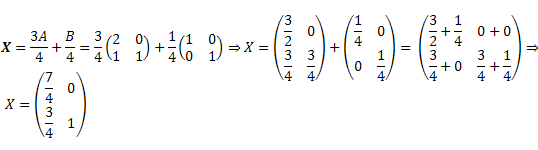
By Gabriel Alessandro de Oliveira
Graduated in Mathematics
Brazil School Team
Matrix and Determinant - Math - Brazil School
Would you like to reference this text in a school or academic work? Look:
OLIVEIRA, Gabriel Alessandro de. "Equations with matrices – Equations involving matrices"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/equacoes-com-matrizesequacoes-matriciais.htm. Accessed on June 29, 2021.