As explained in the text Addition Reactions, these organic reactions usually occur with compounds that have unsaturation (double bonds or triples), in which the pi bond is broken, allowing the entry of atoms or groups of atoms into the chain. carbonic.
However, this type of reaction also occurs in the case of cycloalkanes (closed chain hydrocarbons with only saturated (simple) bonds between carbons) that have three or four carbon atoms. Note an example below, which is the bromination (halogenation reaction) of cyclopropane:
CH2
/ \ + br2 → br CH2 CH2 CH2 ─ br
H2C CH2
Likewise, there is also the addition reaction called hydrohalogenation or the addition of halide, as shown below:
CH2
/ \ + HBr → H CH2 CH2 CH2 ─ br
H2C CH2
Note that, in both cases, the molecule was broken and open-chain compounds were produced.
But this does not happen as easily in cycloalkanes with five or more carbon atoms. On the other hand, these compounds are more likely to perform substitution reactions, in which the bond is not broken, but rather one or more hydrogen atoms bonded to carbon are replaced by atoms of other elements.
Cyclopentane can still carry out addition reactions, but only at higher temperatures (around 300°C). In the case of cyclohexane, this is very difficult. What it actually does are replacement reactions, such as the following chlorination:
CH2 CH2
/ \ / \
H2C CH2 H2C CH ─ Cl
│ │ + Cl2→ │ │ + HCl
H2C CH2 H2C CH2
\ / \ /
CH2 CH2
Rings with five or more carbon atoms do not react with hydrohalic acids, such as HBr, in addition reactions.
But why does this happen? Why do three- or four-carbon cycloalkanes carry out addition reactions and cycloalkanes with more carbon atoms tend not to?
Well, that's because cyclopropane and cyclobutane are more unstable, so it's easier to break their bonds.
Johann Friedrich Adolf von Bayer (1835-1917)
To explain this, the German chemist Johann Friedrich Adolf von Bayer (1835-1917) developed, in 1885, the so-called Ring stress theory, which showed that the four bonds made by the carbon atoms would be more stable when they had an angle equal to 109º 28', as is the case with the following methane:

The four single bonds of methane have an angle of 109º 28'
This is the most stable angle because it corresponds to the greatest possible distance between atoms in a tetrahedral geometry. With this, the electronic repulsion (repulsion between the electrons in the valence layers of the atoms) gets smaller.
Cycloalkanes with three, four and five carbons have bond angles between carbons less than 109º 28'. Look:
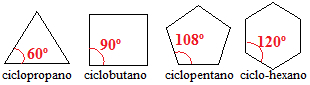
Angles of cycloalkane bonds
Based on these real angles, which we can generically call α, the bond stress calculation can be performed using the following formula:
tension = 109º 28' - α
2
We know that cyclopropane is the most unstable and also the most reactive cycloalkane, and this is confirmed by calculating the voltage of its ring compared to the others:
cyclopropane voltage = 109º 28' – 60º = 109º – 60º + 28' = 49º + 28' = 24,5º + 14
2 2 2
As 0.5º = 30, then we have:
cyclopropane voltage = 24º + 30' + 14' = 24º 44'
cyclobutane voltage = 109º 28' – 90º = 9º 44'2
cyclopentane voltage = 109º 28' – 108º = 0º 44'2
According to Bayer's theory of tensions, the greater this tension, the more unstable the cyclan will be, that is, the greater the difference between the real angle (α) and the theoretical angle (109º 28'), more unstable and, consequently, more reactive will be the substance.
That's why cyclopropane is the least stable of the cycloalkanes.
However, there was an error in Bayer's theory, because if we keep doing this stress calculation for cyclohexane, where the connection angle is 120°, we will see that the value will be even smaller than that of cyclopropane, giving equal to -5° 16'. This would point to the fact that cyclohexane should be even more unstable and carry out addition reactions, which is not the case in practice.
The explanation for this fact was found, in 1890, by the German chemist Hermann Sachse and proved, in 1918, by the also German chemist Ernst Mohr. According to these scientists, the error in Bayer's ring stress theory would lie in the fact that he considered that all cycloalkanes are coplanar, that is, all their carbon atoms are in a single plane, com the drawings of their structures shown above.
However, in reality, the rings of cycloalkanes with more than five carbon atoms are not flat, but their atoms. acquire spatial conformations that cancel the tensions between the connections, establishing an angle of 109º 28' between the Connections.
For example, look at the case of cyclohexane. It is not, in fact, flat with a 120° angle between its bonds, but, in fact, its atoms "twirl", forming two possible conformations, the "chair" and the "boat" conformation:
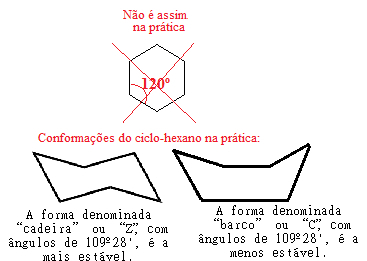
Possible conformations of cyclohexane in practice
Note that, because the real angle of cyclohexane is equal to 109º 28', it is a very stable compound, so its molecule does not break, thus not participating in the addition reactions. Also note that the "chair" shape is the most stable, being the one that always predominates in mixtures, this because, in this conformation, the hydrogen atoms bonded to the carbon are farther apart from each other. others.
By Jennifer Fogaça
Graduated in Chemistry
Source: Brazil School - https://brasilescola.uol.com.br/quimica/teoria-das-tensoes-dos-aneis-bayer.htm

