Study with the Greatest Common Divisor (CDM) exercises and answer your questions with detailed step-by-step resolutions.
question 1
Calculate the MDC between 180 and 150.
To calculate the MDC between 180 and 150, we must perform the decomposition into prime factors and multiply those that simultaneously divide the two columns.

Note that the numbers in red represent the divisors that must be multiplied to determine the MDC. These split numbers into the two columns simultaneously.
Therefore, the Greatest Common Divisor between 180 and 150 is 30.
question 2
Joana is preparing candy kits to distribute among some guests. There are 36 brigadeiros and 42 little cashews. She wants to separate them into dishes in order to occupy the least amount of dishes, but that all dishes have the same amount of sweets and without mixing them. The amount of sweets Joana should put on each plate will be
a) 21.
b) 12.
c) 6.
d) 8.
e) 5.
Correct answer: c) 6.
To find the least amount of dishes to use, it will be necessary to put the greatest amount of sweets in each dish, but making sure that all the dishes have the same amount of sweets and, without mixing brigadeiros and little cashews.
For this, it is necessary to find the greatest common divisor between 36 and 42. Factoring in:
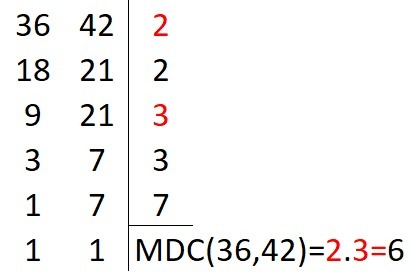
The amount of sweets in each dish will be 6 sweets.
question 3
A team race event will take place next weekend and the registration period for participants ended today. In total, 88 people signed up, 60 women and 28 men. For both modalities, women's and men's, teams must always have the same and as many athletes as possible without mixing men and women in the same team. In this way the number of athletes in each team will be
a) 10.
b) 8.
c) 6.
d) 4.
e) 2.
Correct answer: d) 4.
To know as many athletes as possible on each team, so that they all have the same number of athletes, without mixing men and women in the same team, we must divide the number of entries, men and women, by the Greatest Common Divider between both.
To determine the MDC(28,60), we do factorization.

Entrance exams and competitions issues
question 4
(Post Office – Cespe). The floor of a rectangular room, measuring 3.52 m × 4.16 m, will be covered with square tiles, of the same dimension, whole, so that there is no empty space between neighboring tiles. The tiles will be chosen so that they are as large as possible.
In the situation presented, the side of the tile should measure
a) more than 30 cm.
b) less than 15 cm.
c) more than 15 cm and less than 20 cm.
d) more than 20 cm and less than 25 cm.
e) more than 25 cm and less than 30 cm
Correct answer: a) more than 30 cm.
Note that the question data are in meters and the answers are in centimeters. So let's pass the question values to centimeters.
3.52 m = 352 cm
4.16 m = 416 cm
As the floor is square, all sides must have the same measurement. Therefore, the side measurement must be a common divisor for 352 and 416.
Let's determine the Greatest Common Divisor at 352 and 416.
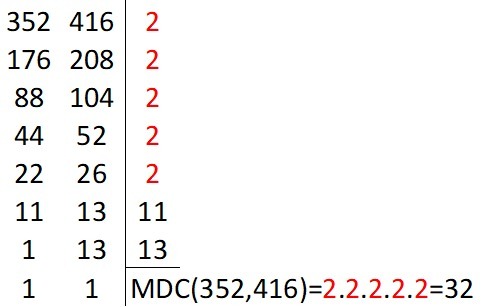
Thus, the answer is the letter a, the tile should measure more than 30 cm.
question 5
(Mathematics Teacher of Basic Education - 2019) A blacksmith will make pieces of iron bars of the same size. It has 35 bars of 270 cm, 18 of 540 cm and 6 of 810 cm, all of equal width. He intends to cut the bars into pieces of the same length, without leaving any leftovers, so that these pieces are as large as possible, but less than 1 m in length. How many pieces of iron rod can the blacksmith produce?
a) 89.
b) 178.
c) 267.
d) 524.
e) 801.
Correct answer: c) 267.
The length of the new pieces should exactly divide the bars already available, so that they are all the same and the longest in length but less than 1 m.
For this, we must factor the measures.

The MDC is 270 cm. However, it is necessary that the new pieces are smaller than 100 cm.
If we remove factor 2, and multiply those that remained highlighted in the factorization, we would have:
3.3.3.5 = 135 cm, even larger than 100 cm.
Removing a factor 3, and multiplying those that remained highlighted in the factorization, we would have:
2.3.3.5 = 90 cm
Therefore, the new pieces must have 90 cm. To find the amount, we must divide each measure of bar already available by 90 and multiply by the amounts of each.
As there are 35 bars of 270, we do the multiplication:
As there are 18 bars of 540, we do the multiplication:
As there are 18 bars of 540, we do the multiplication:
Adding the individual quantities 105 + 108 + 54 = 267.
Therefore, iron the blacksmith can produce 267 pieces of iron bar.
question 6
(Prefeitura de Areial Professor B - Mathematics 2021) The manager of an electronics store, In love with mathematics, he proposes that the price of a certain cell phone be given in reais by the expression mdc (36,42). mmc (36.42).
In this case, it is CORRECT to state that the value of the cell phone, in reais, is equal to:
a) BRL 1,812.00
b) BRL 1,612.00
b) BRL 1,712.00
d) BRL 2,112.00
e) BRL 1,512.00
Correct answer: e) R$ 1,512.00.
First let's calculate the MDC(36,42).
To do this, just factor the numbers and multiply the factors that simultaneously divide the two columns.
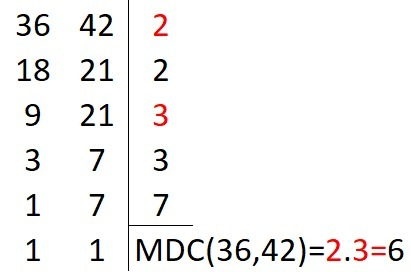
To calculate the MMC, we just multiply all the factors.

Now, just multiply the two results.
252. 6 = 1512
The value of the cell phone, in reais, is equal to R$ 1512.00.
question 7
(Irati Prefecture - SC - English Teacher) In a box, there are 18 blue balls, 24 green balls and 42 red balls. Marta wants to organize the balls into bags, so that each bag has the same number of balls and each color is evenly distributed in the bags and that you can use the maximum amount of bags possible to that. What is the sum of the blue, green and red balls left in each bag?
a) 7
b) 14
c) 12
d) 6
Correct answer: b) 14.
First, let's determine the greatest common divisor of the three numbers;
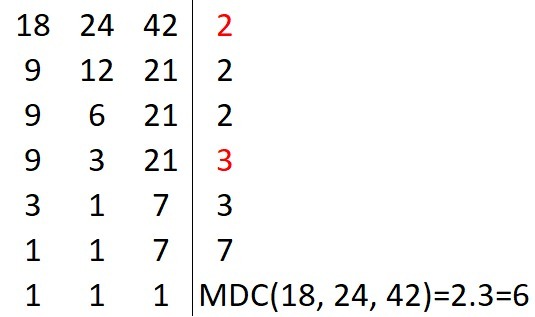
Now, just divide the amount of balls of each color by 6 and add the result.
question 8
(USP-2019) Euler's E function determines, for each natural number ݊n, the amount of natural numbers smaller than ݊n whose greatest common divisor with ݊n is equal to 1. For example, E (6) = 2 since numbers less than 6 with such a property are 1 and 5. What is the maximum value of E (n), for ݊n from 20 to 25?
a) 19
b) 20
c) 22
d) 24
e) 25
Correct answer: c) 22.
E(n) is a function that gives the number of times the MDC between the number n, and a natural number less than n, is equal to 1.
We must determine for n between 20 and 25, which one returns E(n) greater.
Remember that prime numbers are only divisible by 1 and by themselves. Therefore, they are the ones that will have E (n) greater.
Between 20 and 25, only 23 is a prime number. Since E (n) compares the MDC between n and a number smaller than n, we have that E (23) = 22.
Therefore, the maximum value of E (n), for ݊n from 20 to 25, occurs for n=23, where: E(23) = 22.
Just to improve understanding:
MDC(1.23)=1
MDC(2,23)=1
.
.
.
MDC(22.23)=1
question 9
(PUC-PR Medicina 2015) An intern was given the task of organizing documents into three files. In the first file, there were only 42 lease agreements; in the second file, only 30 purchase and sale contracts; in the third file, only 18 property appraisal reports. He was instructed to place documents in folders so that all folders must contain the same amount of documents. In addition to not being able to change any document from its original file, it should put it in the least amount of folders possible. The minimum number of folders it can use is:
a) 13.
b) 15.
c) 26.
d) 28.
e) 30.
Correct answer: b) 15.
We calculate the MDC(18,30,42)

Now we divide the quantities of documents in each file by 6 and add up the result.
So 15 is the minimum number of folders he can use.
exercise more with MMC and MDC - Exercises.
You can also learn more from:
MDC - Maximum Common Divider
MMC and MDC
dividers
Multiples and Dividers