O trigonometric circle it is a circle which has radius 1 and center O. This center is placed at the point O = (0,0) of a Cartesian plane. every point of this circumference is associated with a real number, usually expressed as a function of π, which, in turn, relates to a angle of that circle. As this circle has radius 1, its length is equal to 2π, because:
C = 2πr
C = 2π·1
C = 2π
This real number represents a complete lap. Therefore, the half-turn length in the circletrigonometric can be obtained as follows:
Ç = 2π
2 2
Ç = π
2
As you can see, half-turn has a length equal to π. In the same way, it is possible to show that a quarter of return it has a length equal to π/2 and that three quarters of a turn has a length equal to 3π/2. The location of the points A = π/2, B = π, C = 3π/2 and D = 2π can be seen in the image below. Note that the sense of return given is counterclockwise.
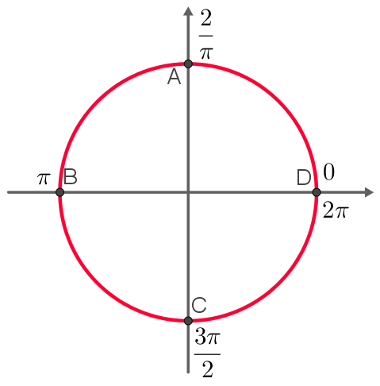
quadrants
The values given for the previous figure mark the divisions of the circletrigonometric in quadrants. Those
quadrants they are also arranged counterclockwise and are numbered by Roman numerals I to IV. The ranges that belong to each quadrant are:1st Quadrant: 0 to π/2;
2nd Quadrant: π/2 to π;
3rd Quadrant: π to 3π/2;
4th Quadrant: 3π/2 to 2π.
These quadrants also support angles. Look:
1st Quadrant: 0 to 90°;
2nd Quadrant: 90° to 180°;
3rd Quadrant: 180° to 270°;
4th Quadrant: 270° to 360°.
Example
The number π/3 is in which quadrant and represents which angle?
From the above, π/3 is in the first quadrant. Knowing that π represents a half turn, that is, 180°, to find the angle represented by π/3, just divide 180° by 3. The result is 60°.
ReasonSine
On a circletrigonometric, construct the angle θ as indicated in the following figure:
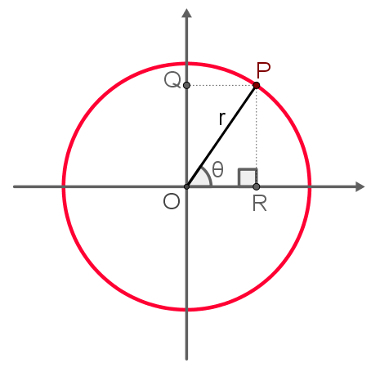
Note that by making the orthogonal projection of P on the x-axis, we get the point R and a right triangle. Making the orthogonal projection of P on the y axis, we get a parallelogram QPR. Calculating the sine of θ, in this case, is equivalent to measuring the length of the segment PR, which is equal to OQ. This is because the damn circle is 1 and the hypotenuse of the triangle in question is always equal to the radius of the circle. Mathematically, we have:
Senθ = PR = PR = PR = OQ
r 1
Note, therefore, that sin0° = 0, sin90° = 1, sin180° = 0 and sin270° = – 1.
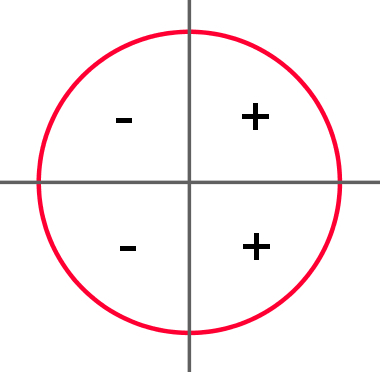
At the circletrigonometric, the sine signs of angle θ can be predicted according to the quadrant in which point P lies. The following figure contains a positive or negative sign for the respective quadrants where sine values are positive or negative.

Reasoncosine
Like cosine the same thing happens, however, the value of the cosine is determined by the length of the segment OR = QP, since the cosine is the result of the division of the adjacent leg by the hypotenuse. Mathematically, we have:
Cosθ = OR = OR = QP
r 1
watching the circletrigonometric, we can identify the main cosine values: Cos0° = 1, Cos90° = 0, Cos 180° = – 1 and Cos 270° = 0. As with sines, it is possible to know the sign of the cosine of the angle in question just by the quadrant that P occupies. Look at the image below:
Example
At the circletrigonometric, mark the sine of 30° and find its value.
Solution:
To solve this problem, construct a 30° angle as follows:

After that, use a ruler to measure the OQ segment or calculate the value of sen30°.
By Luiz Paulo Moreira
Graduated in Mathematics
Source: Brazil School - https://brasilescola.uol.com.br/o-que-e/matematica/o-que-e-circulo-trigonometrico.htm
