Polygons are flat geometric figures formed by straight segments which are connected at their ends in such a way as to form a closed figure and there is no crossing between them. Between the elements of a polygon, are the diagonals, which are straight lines connecting two non-consecutive vertices. Note the following figure which illustrates a “non-polygon” and a polygon.

Elements of a polygon
Sides: are the straight segments that form the polygon;
Vertices: are the meeting points between two sides of a polygon;
diagonals: are the straight lines that connect two non-consecutive points in a polygon;
Interior Angles: Angles that lie within a polygon.

The image illustrates all the elements of a polygon
Number of polygon diagonals
The quadrilaterals are the first polygons that have diagonals. This is because the triangles they only have consecutive vertices. Note the two diagonals of the following square:

The pentagons have five sides and five diagonals different.

Example of a pentagon with its five diagonals
Hexagons have six sides and ninediagonals.
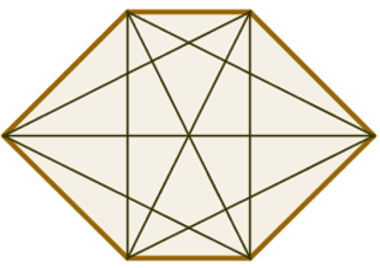
Example of hexagon with its nine diagonals
When the geometric figure has a relatively small number of sides, it is possible to count its diagonals easily. However, when the number of sides of the polygon is high, the task of counting your diagonals it's tiring. For this, there is a formula in which it is enough to replace the letter n by the number of sides of a polygon to find its number of diagonals. This formula is:
D = n (n - 3)
2
*n is the number of sides of the polygon and D is the number of diagonals.
How many diagonals own the pentagon? We already know that there are five diagonals, however, we will use the formula to check this information.
D = n (n - 3)
2
D = 5(5 – 3)
2
D = 5(2)
2
D = 10
2
D = 5
Now let's calculate the number of diagonals of a polygon that has 100 sides.
D = n (n - 3)
2
D = 100(100 – 3)
2
D = 100(97)
2
D = 9700
2
D = 4850
Therefore, a polygon that has 100 sides has 4850 diagonals.
By Luiz Paulo Moreira
Graduated in Mathematics
Take the opportunity to check out our video lesson on the subject: