O termgeneral (Theno) of a arithmetic progression (PA) is a formula used to determine an element of this progression when we know the position (n) of this element, the first term (a1) and the reason (r) of the BP. This formula is:
Theno = the1 + (n – 1)r
To find the formula for termgeneral gives progressionarithmetic, we will give an example, using a PA, of how the terms of this sequence they can be written in terms of the first term and its reason for later doing the same with any PA.
Lookalso: real numbers
Reason and first term of a PA
One arithmetic progression is a numerical sequence in which any element is the result of the sum of its successor with a constant called reason. In other words, the difference between two consecutive terms in an AP is always equal to a constant. The first term, obviously, has no predecessor, so it cannot be the result of the sum of the previous one with reason.
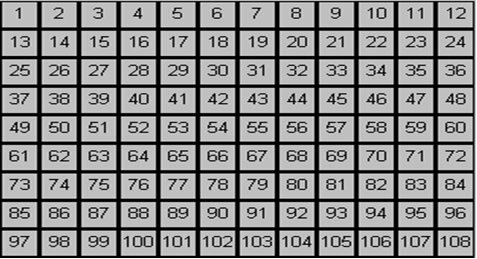
With this in mind, note the following PA elements:
The1 = 10
The2 = 13
The3 = 16
The4 = 19
…
THE reason of this PA is 3, and its first element is 10. We can write all its elements as a result of the first summed with the ratio given number of times. Watch:
The1 = 10
The2 = 10 + 3
The3 = 10 + 3 + 3
The4 = 10 + 3 + 3 + 3
…
Note that the number of times the reason is added to firstterm is always equal to the index of the BP term minus 1. For example, the3 = 10 + 3·2 = 10 + 3·(3 – 1). In this example, the index is 3, and the number of times we add the ratio is 3 – 1 = 2. In this way, we can write:
The1 = 10 + 0·3
The2 = 10 + 1·3
The3 = 10 + 2·3
The4 = 10 + 3·3
…
So, to find the twentieth term of this PA, we can do:
The20 = 10 + 3·(20 – 1)
The20 = 10 + 3·19
The20 = 67
General term of the PA
Using the same reasoning, but with any PA, we can determine the formula of termgeneral of the PA. For this, consider the PA any of the terms:
(The1, a2, a3, a4, a5, …)
Knowing that each element is equal to the first plus the product of the reason for the position of this element minus 1, we can write:
The1 = the1
The2 = the1 + r
The3 = the1 + 2r
The4 = the1 + 3r
…
We can conclude that the term ano of this PA is given by:
Theno = the1 + (n – 1)r
Example
Determine the hundredth term of the BP: (1, 7, 14, 21, …).
Using the formula of termgeneral, we will have:
Theno = the1 + (n – 1)r
The100 = 1 + (100 – 1)7
The100 = 1 + (99)7
The100 = 1 + 693
The100 = 694
Take the opportunity to check out our video lesson on the subject: