Numbers are always present in our lives and there is no way to escape them for a single second. The creation of numbers arose from the natural need of human beings to count the members of their group, the animals in their herds and their collections of objects. When the human being stopped being a nomad and began to domesticate animals for food, the need to count led him to the path of numbers. Since then, numbers have fascinated many people, especially mathematicians.
Pythagoras was one of the most famous Greek mathematicians who studied, in addition to geometry, numbers. As Pythagoras was always curious when it came to geometry, he tried to establish relationships between numbers and flat figures. With his studies, he realized that there was even a connection between numbers and geometry and ended up discovering the triangular numbers and the square numbers.
Triangular numbers are those that can be represented in the shape of a triangle. Note the sequence below:
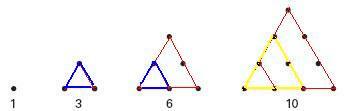
With the number of dots representing each number, Pythagoras observed that a triangle could be constructed. Can you determine what is the next triangular number after 10?
Quadrangular numbers are, like the previous ones, numbers that can represent a square shape. See the picture:
And the next two numbers in that sequence, are you able to find out?
See that Pythagoras found a fun way to deal with numbers, drawing, looking for relationships with other areas of mathematics and other sciences. Math can be fun and interesting.
How about doing like Pythagoras and trying to find other numbers that can become a figure? Draw with your friends, challenge them to find the next numbers in each of the sequences above. Have fun with math!
*The next triangular number after 10 is 15. And the next two square numbers after 16 are 25 and 36.
By Marcelo Rigonatto
Mathematical
Kids School Team