The current financial market offers various credit operations for those who want to finance a car, real estate, build their own business, invest in the company, among other options. Financial institutions offer a capital that must be returned with interest during a pre-determined period. The ways to pay off the loan are numerous, let's talk about the functioning of the amortization system constant, which consists of the payment of the debt based on installments of equal amortization with installments and interest decreasing. To better understand the SAC, let's build a detailed table involving a given situation.
Example 1
A bank releases a credit of R$ 120,000.00 to a person to be paid by the SAC in 10 monthly installments. Since the interest rate is 5% per month, build the spreadsheet.
Calculating the amortization amount:
120 000 / 10 = 12 000
Monthly amortizations will be fixed and equal to R$ 12,000.00
Do not stop now... There's more after the advertising ;)
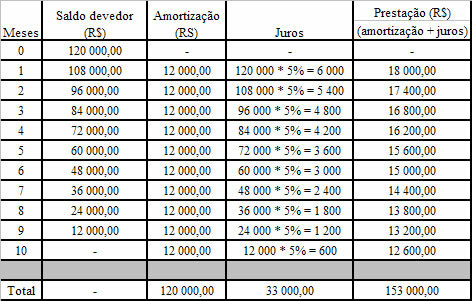
Note that interest is calculated on the amount of the previous month's outstanding balance, and the installments are obtained by adding the interest for the period and the repayment amount.
Example 2
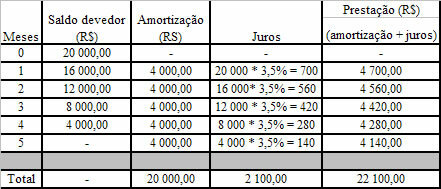
A loan in the amount of R$20,000.00 must be paid by the SAC in 5 monthly installments with a monthly interest of 3.5%. Build the payment sheet for that debt.
Determining the amount of amortizations:
20 000 / 5 = 4 000
The constant amortizations will be BRL 4,000.00
by Mark Noah
Graduated in Mathematics
Brazil School Team
Financial math - Math - Brazil School
Would you like to reference this text in a school or academic work? Look:
SILVA, Marcos Noé Pedro da. "SAC: Constant Amortization System"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/sac-sistema-amortizacoes-constantes.htm. Accessed on June 29, 2021.