Integrate means to determine the primitive function in relation to a previously derived function, that is, we will perform an inverse operation of the derivation. We call a function F(x) of primitive f(x) at a given interval, only if for all I we have F’(x) = f(x).
If F(x) is an integral of f(x), then F(x) + C is also, C being an arbitrary constant. For example, the functions given by x², x² + 6, x² - 2 and x² + 10 are integrals of 2x, given that d/dx (x²) = d/dx (x² + 6) = d/dx (x² - 2) = d/dx (x² + 10) = 2x.
To perform the function integrations, aiming to discover the primitive function, we use some fundamental integration formulas. Watch:
1. ∫ d/dx [f (x)] dx = f (x) + C
2. ∫(u + v) dx = ∫ u dx + ∫ v dx
3. ∫ au dx = a ∫ u dx, where a is any constant.
4. uno du = ∫ (un+1/n+1) + C, if n ≠ – 1
5. ∫ du/u = ln u + C, if u > 0
6. tou du = au/lna + C, if a > 0
7. ∫ andu du = andu + C
8. ∫ sin u du = – cos u + C
Do not stop now... There's more after the advertising ;)
9. ∫ cos u du = sin u + C
10. ∫ tg u du = ln sec u + C
11. ∫ cotg u du = ln sin u + C
12. ∫ sec u du = ln (sec u + yg u) + C
13. ∫ cosec u du = ln (cosec u – cotg u) + C
14. ∫ sec² u du = tg u + C
15. ∫ cosec² u du = – cotg u + c
16. ∫ sec u tg u du = sec u + C
17. ∫ cosec u cotg u du = – cosec u + C
18. 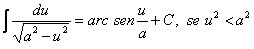
19.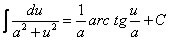
20. 
21. 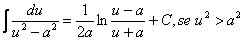
22. 
23. 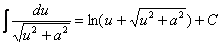
24. 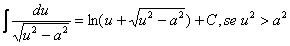
25. 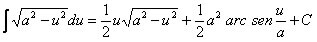
26. 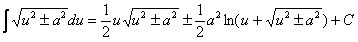
by Mark Noah
Graduated in Mathematics
Brazil School Team
Occupation - Math - Brazil School
Would you like to reference this text in a school or academic work? Look:
SILVA, Marcos Noé Pedro da. "Fundamental Integration Formulas"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/formulas-fundamentais-integracao.htm. Accessed on June 29, 2021.