Trigonometric functions of the half arc

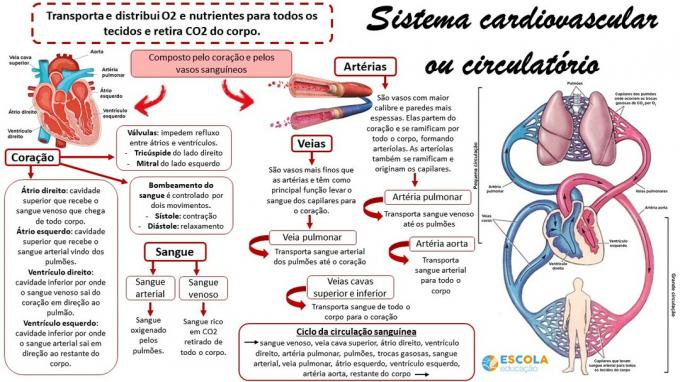
At trigonometric functions, sine, cosine and tangent, of the arc half can be obtained from the trigonometric functions of the double arc.

Given an arc of measure $\dpi{120} \alpha$ , the double bow is the bow $\dpi{120} 2\alpha$ and the half bow is the bow $\dpi{120} \alpha/2$ .

By two arc addition formulas, we have the trigonometric functions of the double arc:

Sine:

$\dpi{120} \mathrm{sen (2{\alpha})=sen({\alpha + \alpha}) = sin\, {\alpha} \cdot cos\, {\alpha} + sin\, {\ alpha} \cdot cos\, {\alpha}}$

$\dpi{120} \Rightarrow \mathbf{sen (2\boldsymbol{\alpha})= 2. (sen\, \boldsymbol{\alpha} \cdot cos\, \boldsymbol{\alpha}) }$

cosine:

$\dpi{120} \mathrm{cos (2{\alpha})=cos({\alpha + \alpha}) = cos\, {\alpha} \cdot cos\, {\alpha} - sin\, {\ alpha} \cdot sin\, {\alpha}}$

$\dpi{120} \Rightarrow \mathbf{cos (2\boldsymbol{\alpha})= cos^2\, \boldsymbol{\alpha} - sen^2\, \boldsymbol{\alpha} }$

Tangent:

$\dpi{120} \mathrm{tan (2{\alpha})=tan({\alpha + \alpha}) = \frac{tan\, {\alpha} + tan\, {\alpha}}{1 - tan\, {\alpha} \cdot tan\, {\alpha}}}$

$\dpi{120} \Rightarrow \mathbf{tan (2\boldsymbol{\alpha})= \frac{2\cdot tan\, \boldsymbol{\alpha} }{1 - tan^2\, \boldsymbol{\alpha }}}$

From these formulas, we will show the formulas of the half arc trigonometric functions.

Trigonometric functions of the half arc

One of fundamental relations of trigonometry is that:

$\dpi{120} \mathbf{sen^2\boldsymbol{\alpha} + cos^2\boldsymbol{\alpha} = 1}$

Where do we get:

$\dpi{120} \mathrm{sen^2\alpha = 1 - cos^2\alpha}$

$\dpi{120} \mathrm{ cos^2\alpha = 1-sen^2\alpha }$

replacing $\dpi{120} \mathrm{sen^2\alpha = 1 - cos^2\alpha}$ in the formula of the cosine of the double arc, we have to:

$\dpi{120} \mathrm{cos (2{\alpha})= cos^2\, {\alpha} - sin^2\, {\alpha} = cos^2\, {\alpha} - (1 - cos^2\, {\alpha})}$

Check out some free courses

Free Online Inclusive Education Course
Free Online Toy Library and Learning Course
Free Online Math Games Course in Early Childhood Education
Free Online Pedagogical Cultural Workshops Course

$\dpi{120} \mathrm{= 2cos^2\, {\alpha} - 1 }$

Therefore: $\dpi{120} \mathrm{cos (2\alpha)= 2cos^2\, {\alpha} - 1 }$

$\dpi{120} \Rightarrow \mathrm{cos^2\, {\alpha} =\frac{1+cos (2\alpha) }{2} }$

replacing $\dpi{120} \alpha$ per $\dpi{120} \alpha/2$ in the formula above and extracting the square root on both sides, we have the formula for cosine of arc half:

$\dpi{120} \mathbf{cos\, {(\boldsymbol{\alpha}/2)} = \pm \sqrt{\frac{1+cos\, \boldsymbol{\alpha} }{2} }}$

Note: The sign in the formula will be positive or negative according to the quadrant of the arc half.

instagram story viewer

Now replacing $\dpi{120} \mathrm{ cos^2\alpha = 1-sen^2\alpha }$ in the formula of the cosine of the double arc, we have to:

$\dpi{120} \mathrm{cos (2{\alpha})= cos^2\, {\alpha} - sin^2\, {\alpha} = (1 -sen^2\, {\alpha}) - sen^2\, {\alpha} }$

$\dpi{120} \mathrm{= 1-2sen^2\, {\alpha} }$

Therefore:

$\dpi{120} \mathrm{cos (2\alpha)= 1-2sen^2\, {\alpha} }$

$\dpi{120} \Rightarrow \mathrm{sen^2\, {\alpha} =\frac{1-cos (2\alpha)}{2} }$

replacing $\dpi{120} \alpha$ per $\dpi{120} \alpha/2$ in the formula above and extracting the square root on both sides, we have the formula for sine of arc half:

$\dpi{120} \mathbf{sen\, {(\boldsymbol{\alpha}/2)} = \pm \sqrt{\frac{1-cos\, \boldsymbol{\alpha}}{2}} }$

Note: The sign in the formula will be positive or negative according to the quadrant of the arc half.

Finally, we can obtain the tangent of the arc half, dividing the sine of the arc half by the cosine of the arc half:

$\dpi{120} \mathrm{tan(\alpha/2) = \frac{sen(\alpha/2)}{cos(\alpha/2)} = \frac{\sqrt{\frac{1 - cos\, \alpha}{2}}}{\sqrt{\frac{1 + cos\, \alpha}{2}}} = \sqrt{\frac{1 - cos\, \alpha}{1 + cos\, \alpha}}}$

Therefore, the formula of half arc tangent é:

$\dpi{120} \mathbf{tan(\boldsymbol{\alpha}/2) = \pm \sqrt{\frac{1 - cos\, \boldsymbol{\alpha}}{1 + cos\, \boldsymbol{\ alpha}}}}$

Note: The sign in the formula will be positive or negative according to the quadrant of the arc half.

You may also be interested:

trigonometric circle
trigonometric table
Trigonometric ratios
sins law
cosine law

The password has been sent to your email.

Trigonometric functions of the half arc

Trigonometric functions of the half arc

Parts of the human body

Calculation of slope

Exercises on reason and proportion