The concepts of reversible and irreversible processes can be described mathematically using the concept of entropy. But before we get to the definition of entropy, let's get to the concepts of reversible and irreversible processes. we call reversible process one in which the system can spontaneously return to the original situation (or state). Irreversible process is one whose system cannot spontaneously return to its original state.
As the concepts of the types of processes have already been mentioned, let's go to the definition of entropy. THE entropy of a system (S) is a measure of its degree of disorganization. The larger the organization, the lower the entropy. Entropy is a characteristic of the thermodynamic state, as are internal energy, volume, and the number of moles.
Looking at the containers in the figure above, we see that container 1 has a entropy smaller than the other. If we take the container and shake it, we will verify that the “balls” will be mixed up, or rather, disorganized. If we check container 2, after shaking it, we will notice that it is not possible for the balls, spontaneously, return to their original organization, if we continue to shake the container.
Do not stop now... There's more after the advertising ;)
In reversible isothermal (whose temperature always remains the same) reversible processes, we define entropy as the ratio of heat (given or received) to temperature. Thus, we represent the entropy in isothermal processes as follows:
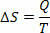
In the International System of Units, we measure entropy in joule/kelvin. Based on the concept we describe about entropy, we can formulate the Second Law as follows:

The entropy change of an isolated system is always positive or null. The equality ΔS = 0 occurs when processes are reversible: reversible processes do not increase entropy. Isolated systems, which neither receive nor give heat to the environment, can only have their entropy increased or kept constant.
By Domitiano Marques
Graduated in Physics
Brazil School Team
Would you like to reference this text in a school or academic work? Look:
SILVA, Domitiano Correa Marques da. "Entropy and the Second Law"; Brazil School. Available in: https://brasilescola.uol.com.br/fisica/entropia-segunda-lei.htm. Accessed on June 27, 2021.