THE definition of circle is closely linked to the definition of circle. One circle is a set of points resulting from the union of a circle with all its internal points. Thus, when filling a circular pool of water, for example, the edge of that pool and the surface of the water form a circle.
A circle, in turn, is a set of points on the plane equidistant from another fixed point on the same plane.. This means that, given a fixed point C (a point that remains in the same place, without moving), any point that has a distance r from point C belongs to the circle.
To build a circle, just take a string of length r, fix one of its ends to a fixed point and, with the free end of the rope, trace the curve formed by a movement that keeps it taut. If the string is not taut, the distance between its ends will be less than r. The figure obtained from this experience would be as follows:

Circumference with center C and radius r
Bearing in mind that the circle is a set of points distant from a fixed point, what happens to points that have distances less than r? The answer to this question can be found in the definition of circle:
What is Circle?
Definition of circle: Circle is the union of a circle with all the points inside it.
In other words, the circumference is just the outline of a circle. In this way, the distance between the center and any point on a circle is always less than or equal to r.
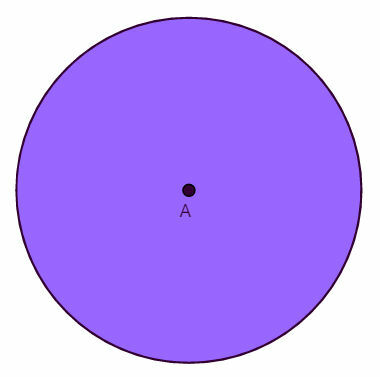
Point A is called the center, the outline, in the same color as point A is the circumference and the interior is the circle.
For the circle, all the radius, diameter and chord properties of a circle apply. In addition to these properties, circles are divided into two sets of equal points, called semicircles, for any diameter.
With respect to points, any point A where the distance from A to O, represented by d (A, O), is equal to the radius is called a point of the circumference. Any point B where d(B, O) is less than the radius is called point inside the circle. In these two cases, the points belong to the circle. Finally, any point C where d(C, O) is greater than the radius is called point outside the circle.
Ancient peoples already knew measurements involving circles and circumferences. Some of them measured a circumference and divided the value found by the length of its diameter. Any attempt at this experiment had a fixed number as a result: approximately 3.14. There have been few attempts at this calculation to note that this value is always found, regardless of the circumference. Thus, where C is the length of the circumference and d its diameter, we have:
Ç = 3,14
d
Knowing that the diameter of a circle is equal to twice its radius (d = 2r), we can substitute the above expression as follows:
Ç = 3,14
2nd
It is now known that the number resulting from this division is an irrational number (with infinitely many decimal places). Therefore, using the Greek letter π (read pi) to represent this number, the formula for calculating the length of a circle is given by:
C = 2.π.r
This is also the formula used to calculate the circle perimeter, since circle perimeter and circumference are the same thing.
About the calculating the area of a circle, it is given by the following expression:
A = π.r2
That said, it is more correct to say that the area calculation is done only on the circle or that the area to be calculated is delimited by a circle. However, it is common to find exercises and problems whose calculation proposals are for the area of the circle.
By Luiz Paulo Moreira
Graduated in Mathematics
Source: Brazil School - https://brasilescola.uol.com.br/o-que-e/matematica/o-que-e-circulo.htm

