As we know, the electric field plays the role of transmitter of interactions between electric charges.
Imagine a point-shaped electrical charge* Q in any region of space. This load modifies the region that surrounds it, so that when we place a test point load q at a point P in this region, the existence of a force F, of an electrical nature, acting on q.
Likewise, the electric charge q produces an electric field that acts on Q.
The strength of the electric field generated by a charge Q can be calculated by the equation:
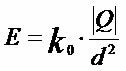
Where:
k0 = 9x109 Nm2/Ç2 (electrostatic constant in vacuum)
Q = generating load of the electric field under study
d = distance between charge Q and point P.
The direction and direction of the electric field depend on the sign of the charge that generates this field.
If Q > 0, the electric field is a distance, and if Q < 0, the electric field is an approximation.
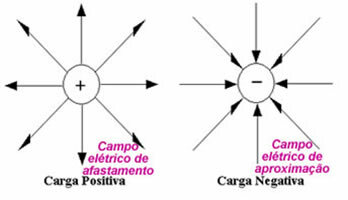
It is common to hear the terms: Field of Attraction and Field of Repulsion, referring to the field of Approximation and Offset field, but that is a wrong notation and should not be used in under no circumstances.
When the electric field is created by several fixed point charges, Q1, Q2,..., QN we can determine the electric field created by these charges at any point P in space.
If Q1 were alone, it would originate in P the field vector
as well as Q2, alone, would originate in P a field vector 
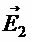and so on, until QN which alone would generate the field vector 
.The resulting electric field vector at point P, due to various charges, is the vector sum of the fields.
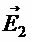, , , where each partial vector is determined as if the respective charge were alone. I.e,
.Example:
Let two charges +Q and -Q be arranged in a vacuum as shown in the figure below:
It is known that the modulus of loads are equal to Q. Therefore, calculate the intensity, direction and direction of the resulting electric field vector in P. Assume that Q = 2.10-6 C and that d = 0.3 m.
Note that charge + Q generates, in P, an electric field vector of REMOVAL.
Also note that the charge –Q generates, in P, an APPROACH electric field vector.
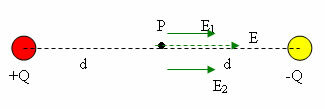
As the charges are equidistant from point P, the electric fields generated by them have the same intensity, direction and direction, thus: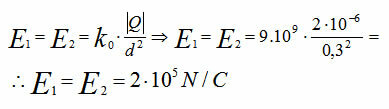
Thus, the intensity of the resulting electric field is:
Its direction is horizontal and the direction is left to right.
* Dot-shaped electrical charge is an electrical charge that has negligible dimensions.
By Kléber Cavalcante
Graduated in Physics
Brazil School Team
Electricity - Physics - Brazil School
Source: Brazil School - https://brasilescola.uol.com.br/fisica/campo-eletrico-gerado-por-varias-cargas.htm
